Page 43 - u4

P2: OSO/OVY
P1: OSO/OVY
GO01962-Smith-v1.cls
UAE_Math_Grade_12_Vol_1_SE_718383_ch3
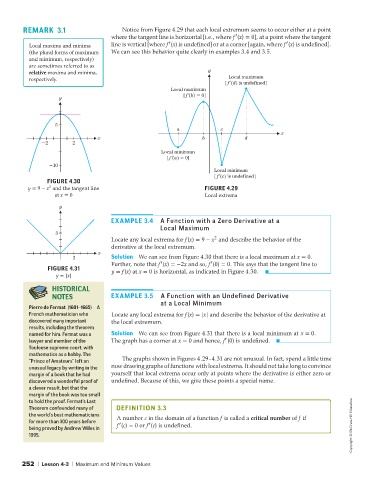
Notice from Figure 4.29 that each local extremum seems to occur either at a point
REMARK 3.1 QC: OSO/OVY T1: OSO July 4, 2016 13:38
′
where the tangent line is horizontal [i.e., where f (x) = 0], at a point where the tangent
′
′
Local maxima and minima line is vertical [where f (x) is undefined] or at a corner [again, where f (x) is undefined].
(the plural forms of maximum We can see this behavior quite clearly in examples 3.4 and 3.5.
and minimum, respectively)
are sometimes referred to as
relative maxima and minima, y
respectively. Local maximum
[ f (d) is undefined]
Local maximum
[ f (b) = 0]
y

5
a c
x
x b d
-2 2
Local minimum
[ f (a) = 0]
-10
Local minimum
[ f (c) is undefined]
FIGURE 4.30
2
y = 9 − x and the tangent line FIGURE 4.29
at x = 0 Local extrema
y
EXAMPLE 3.4 A Function with a Zero Derivative at a
Local Maximum
3
2
Locate any local extrema for f(x) = 9 − x and describe the behavior of the
derivative at the local extremum.
x
2 Solution We can see from Figure 4.30 that there is a local maximum at x = 0.
′
′
Further, note that f (x) =−2x and so, f (0) = 0. This says that the tangent line to
FIGURE 4.31
y = f(x) at x = 0 is horizontal, as indicated in Figure 4.30.
y = |x|
HISTORICAL
NOTES EXAMPLE 3.5 A Function with an Undefined Derivative
at a Local Minimum
Pierre de Fermat (1601–1665) A
French mathematician who Locate any local extrema for f(x) = |x| and describe the behavior of the derivative at
discovered many important the local extremum.
results, including the theorem
named for him. Fermat was a Solution We can see from Figure 4.31 that there is a local minimum at x = 0.
′
lawyer and member of the The graph has a corner at x = 0 and hence, f (0) is undefined.
Toulouse supreme court, with
mathematics as a hobby. The
“Prince of Amateurs” left an The graphs shown in Figures 4.29–4.31 are not unusual. In fact, spend a little time
unusual legacy by writing in the now drawing graphs of functions with local extrema. It should not take long to convince
margin of a book that he had yourself that local extrema occur only at points where the derivative is either zero or
discovered a wonderful proof of undefined. Because of this, we give these points a special name.
a clever result, but that the
margin of the book was too small
to hold the proof. Fermat’s Last
Theorem confounded many of DEFINITION 3.3
the world’s best mathematicians A number c in the domain of a function f is called a critical number of f if
for more than 300 years before ′ ′
being proved by Andrew Wiles in f (c) = 0 or f (c) is undefined. Copyright © McGraw-Hill Education
1995.

252 | Lesson 4-3 | Maximum and Minimum Values