Page 45 - u4

P2: OSO/OVY
QC: OSO/OVY
P1: OSO/OVY
T1: OSO
13:38
July 4, 2016
GO01962-Smith-v1.cls
UAE_Math_Grade_12_Vol_1_SE_718383_ch3
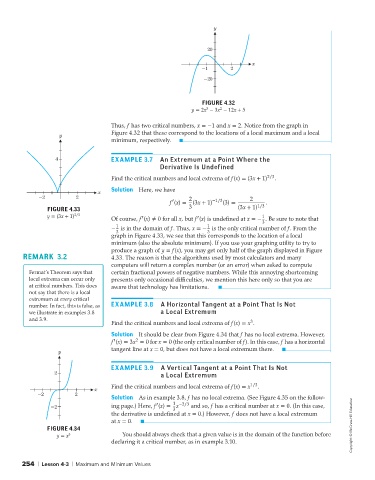
y
20
x
-1 2

-20

FIGURE 4.32
3
2
y = 2x − 3x − 12x + 5
Thus, f has two critical numbers, x =−1 and x = 2. Notice from the graph in
Figure 4.32 that these correspond to the locations of a local maximum and a local
y
minimum, respectively.

4 EXAMPLE 3.7 An Extremum at a Point Where the
Derivative Is Undefined

Find the critical numbers and local extrema of f(x) = (3x + 1) 2∕3 .
Solution Here, we have
x
-2 2 2 2
′
f (x) = (3x + 1) −1∕3 (3) = .
FIGURE 4.33 3 (3x + 1) 1∕3
y = (3x + 1) 2∕3 ′ ′ 1
Of course, f (x) ≠ 0 for all x, but f (x) is undefined at x =− . Be sure to note that
3
1
1
− is in the domain of f. Thus, x =− is the only critical number of f. From the
3
3
graph in Figure 4.33, we see that this corresponds to the location of a local
minimum (also the absolute minimum). If you use your graphing utility to try to
produce a graph of y = f(x), you may get only half of the graph displayed in Figure
REMARK 3.2 4.33. The reason is that the algorithms used by most calculators and many
computers will return a complex number (or an error) when asked to compute
Fermat’s Theorem says that certain fractional powers of negative numbers. While this annoying shortcoming
local extrema can occur only presents only occasional difficulties, we mention this here only so that you are
at critical numbers. This does aware that technology has limitations.
not say that there is a local
extremum at every critical
number. In fact, this is false, as EXAMPLE 3.8 A Horizontal Tangent at a Point That Is Not
we illustrate in examples 3.8 a Local Extremum
and 3.9.
3
Find the critical numbers and local extrema of f(x) = x .
Solution It should be clear from Figure 4.34 that f has no local extrema. However,
′
2
f (x) = 3x = 0 for x = 0 (the only critical number of f). In this case, f has a horizontal
tangent line at x = 0, but does not have a local extremum there.
y
EXAMPLE 3.9 A Vertical Tangent at a Point That Is Not
2 a Local Extremum

Find the critical numbers and local extrema of f(x) = x 1∕3 .
x
-2 2
Solution As in example 3.8, f has no local extrema. (See Figure 4.35 on the follow-
′
1 −2∕3
-2 ing page.) Here, f (x) = x and so, f has a critical number at x = 0. (In this case,
3
the derivative is undefined at x = 0.) However, f does not have a local extremum
at x = 0.
FIGURE 4.34 Copyright © McGraw-Hill Education
y = x 3 You should always check that a given value is in the domain of the function before
declaring it a critical number, as in example 3.10.

254 | Lesson 4-3 | Maximum and Minimum Values