Page 50 - u4

T1: OSO
P2: OSO/OVY
QC: OSO/OVY
P1: OSO/OVY
P1: OSO/OVY
GO01962-Smith-v1.cls
GO01962-Smith-v1.cls
October 22,
UAE_Math_Grade_12_Vol_1_SE_718383_ch4
UAE-Math-Grade-12-Vol-1-SE-718383-ch4
CHAPTER 4 • • • •
Applications of Differentiation
CHAPTER 4
Applications of Differentiation
242
242 P2: OSO/OVY QC: OSO/OVY T1: OSO October 25, 2018 2019 17:24 15:32 4-32
4-32
8'
52. Sketch a graph of f(x) = −x 2 2 and determine where the 8 in
and determine where the
52. Sketch a graph of f(x) = e e
−x
graph is steepest. (Note: This is an important problem in in
graph is steepest. (Note: This is an important problem
probability theory.)
probability theory.)
6'
6 in
A A
APPLICATIONS
APPLICATIONS
53. If two soccer teams each score goals at a rate of r goals 5'
53. If two soccer teams each score goals at a rate of r goals
5 ft
per minute, the probability that n goals will be scored in in
per minute, the probability that n goals will be scored
(rt)
1 1
t minutes is P = = (rt) n n e . Take r = = 25 . Show that for a 90-
−rt
−rt
t minutes is P
e . Take r
. Show that for a 90-
n! n!
25
minute game, P is maximized with n = 3. Briefly explain
minute game, P is maximized with n = 3. Briefly explain
why this makes sense. Find t to maximize the probability
why this makes sense. Find t to maximize the probability
that exactly 1 goal has been scored. Briefly explain why
that exactly 1 goal has been scored. Briefly explain why x x
your answer makes sense.
your answer makes sense. Whatchangesiftheperson’seyesare6feetabovetheground?
Whatchangesiftheperson’seyesare6feetabovetheground?
54. If you have won three out of four matches against some-
54. If you have won three out of four matches against some- 60. Suppose that a hockey player is shooting at a 6-foot-wide
60. Suppose that a hockey player is shooting at a 6-foot-wide
one, does that mean that the probability that you will win net from a distance of d feet away from the goal line and
one, does that mean that the probability that you will win
net from a distance of d feet away from the goal line and
3 3
the next one is ? In general, if you have a probability 4 ft to the side of the center line. (a) Find the distance d that
the next one is ? In general, if you have a probability p p
4 in to the side of the center line. (a) Find the distance d
4 4
of winning each match, the probability of winning m out that maximizes the shooting angle. (b) Repeat part (a) with
of winning each match, the probability of winning m out
maximizes the shooting angle. (b) Repeat part (a) with the
the shooter 2 ft to the side of the center line. Explain why
m m
n−m
of n matches is f(p) = = n! n! p (1 − n−m . Find p shooter 2 ft to the side of the center line. Explain why the
of n matches is f(p)
p (1 − p) p)
. Find p to to
(n − m)!m! answer is so different. (c) Repeat part (a) with the goalie
(n − m)!m!
the answer is so different. (c) Repeat part (a) with the goalie
maximize f. This value of p is called the maximum likeli- blocking all but the far 2 ft of the goal.
maximize f. This value of p is called the maximum likeli-
blocking all but the far 2 ft of the goal.
hood estimator of the probability. Briefly explain why your
hood estimator of the probability. Briefly explain why your
answer makes sense.
answer makes sense.
EXPLORATORY EXERCISES
55. A section of roller coaster is in the shape of of EXPLORATORY EXERCISES
55. A section of roller coaster is in the shape
3 3
5 5
y = x − 4x − x + 10, where x is between −2 and 2. Find −x −x 2 −x 3 −x
y = x − 4x − x + 10, where x is between −2 and 2. Find
−x
and x e . Find all local
−x
1. Explore the graphs of e , xe , x e e
3 −x
2 −x
all local extrema and explain what portions of the roller 1. Explore the graphs of e , xe , x and x e . Find all local
all local extrema and explain what portions of the roller
extrema and use l’Hôpital’s Rule to determine the behavior
coaster they represent. Find the location of the steepest
coaster they represent. Find the location of the steepest extrema and use l’Hôpital’s Rule to determine the behavior
n −x
n −x
as x → ∞. You can think of the graph of x
as showing
part of the roller coaster.
part of the roller coaster. as x → ∞. You can think of the graph of x e e as showing
−x
the results of a tug-of-war: x → ∞ as x → ∞ but −x → 0 0
the results of a tug-of-war: x → ∞ as x → ∞ but e e
n n
→
56. Suppose a large computer file is sent over the Internet. If If
56. Suppose a large computer file is sent over the Internet. as x → ∞. Describe the graph of x n −x in terms of this tug-
n −x
as x → ∞. Describe the graph of x e e
in terms of this tug-
the probability that it reaches its destination without any er-
the probability that it reaches its destination without any er- of-war.
of-war.
rors is x, then the probability that an error is made is 1 −
rors is x, then the probability that an error is made is 1 − x. x.
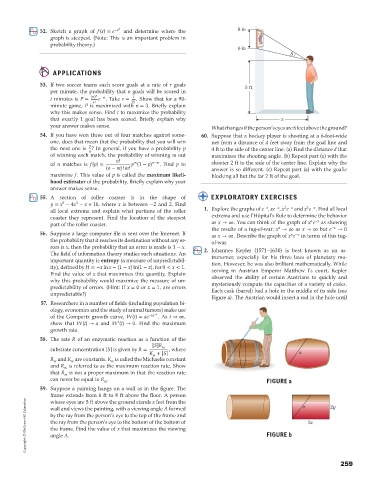
2. Johannes Kepler (1571–1630) is best known as an as-
The field of information theory studies such situations. An 2. Johannes Kepler (1571–1630) is best known as an as-
The field of information theory studies such situations. An
tronomer, especially for his three laws of planetary mo-
important quantity is entropy (a measure of unpredictabil- tronomer, especially for his three laws of planetary mo-
important quantity is entropy (a measure of unpredictabil-
tion. However, he was also brilliant mathematically. While
ity), defined by H =−x ln x − (1 − x) ln(1 − x), for 0 < x < 1. 1. tion. However, he was also brilliant mathematically. While
ity), defined by H =−x ln x − (1 − x) ln(1 − x), for 0 < x <
serving in Austrian Emperor Matthew I’s court, Kepler
Find the value of x that maximizes this quantity. Explain
Find the value of x that maximizes this quantity. Explain serving in Austrian Emperor Matthew I’s court, Kepler
observed the ability of certain Austrians to quickly and
why this probability would maximize the measure of un- observed the ability of certain Austrians to quickly and
why this probability would maximize the measure of un-
mysteriously compute the capacities of a variety of casks.
predictability of errors. (Hint: If x = 0 or x = 1, are errors
predictability of errors. (Hint: If x = 0 or x = 1, are errors mysteriously compute the capacities of a variety of casks.
Each cask (barrel) had a hole in the middle of its side (see
unpredictable?)
unpredictable?) Each cask (barrel) had a hole in the middle of its side (see
Figure a). The Austrian would insert a rod in the hole until
Figure a). The Austrian would insert a rod in the hole until
57. Researchers in a number of fields (including population bi-
57. Researchers in a number of fields (including population bi-
ology, economics and the study of animal tumors) make use
ology, economics and the study of animal tumors) make use
of the Gompertz growth curve, W(t) = −be −t −t . As t → ∞,
of the Gompertz growth curve, W(t) = ae ae
−be
. As t → ∞,
′ ′
show that W(t) → a and W (t) → 0. Find the maximum
show that W(t) → a and W (t) → 0. Find the maximum
growth rate.
growth rate.
58. The rate R of an enzymatic reaction as a function of the
58. The rate R of an enzymatic reaction as a function of the
[S]R
[S]R
, where
substrate concentration [S] is given by R = = m m , where
substrate concentration [S] is given by R
K + [S]
K + [S] z z
m m
R and K are constants. K is called the Michaelis constant
R and K are constants. K is called the Michaelis constant
m m
m m
m m
and R is referred to as the maximum reaction rate. Show
and R is referred to as the maximum reaction rate. Show
m m
that R is not a proper maximum in that the reaction rate
that R is not a proper maximum in that the reaction rate
m m
can never be equal to R m m FIGURE a a
can never be equal to R . .
FIGURE
59. Suppose a painting hangs on a wall as in the figure. The
59. Suppose a painting hangs on a wall as in the figure. The
frame extends from 6 ft to 8 ft above the floor. A person z z 2y
frame extends from 6 ft to 8 ft above the floor. A person
Copyright © McGraw-Hill Education by the ray from the person’s eye to the top of the frame and FIGURE b b 2x
whose eyes are 5 ft above the ground stands x feet from the
whose eyes are 5 ft above the ground stands x feet from the
2y
wall and views the painting, with a viewing angle A formed
wall and views the painting, with a viewing angle A formed
by the ray from the person’s eye to the top of the frame and
the ray from the person’s eye to the bottom of the bottom
the ray from the person’s eye to the bottom of the bottom of of
2x
the frame. Find the value of x that maximizes the viewing
the frame. Find the value of x that maximizes the viewing
FIGURE
angle A. A.
angle
259