Page 53 - u4

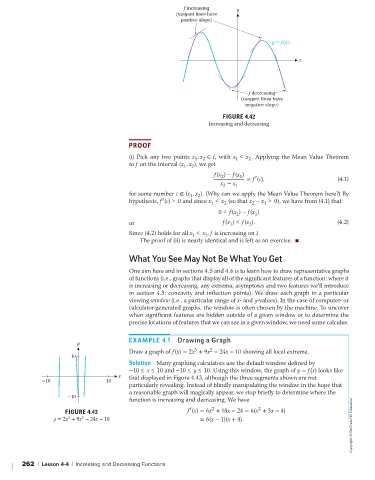
f increasing y
(tangent lines have
positive slope)

y = f(x)

x

f decreasing
(tangent lines have
negative slope)
FIGURE 4.42
Increasing and decreasing

PROOF
(i) Pick any two points x ,x I, with x < x . Applying the Mean Value Theorem
1
2
1
2
to f on the interval (x ,x ), we get
1
2
f(x ) − f(x ) = f (c), (4.1)
1
2
x − x 1
2
for some number c (x ,x ). (Why can we apply the Mean Value Theorem here?) By
2
1
hypothesis, f (c) > 0 and since x < x (so that x − x > 0), we have from (4.1) that
2
2
1
1
0 < f(x ) − f(x )
1
2
or f(x ) < f(x ). (4.2)
2
1
Since (4.2) holds for all x < x ,f is increasing on I.
2
1
The proof of (ii) is nearly identical and is left as an exercise.
What You See May Not Be What You Get
One aim here and in sections 4.5 and 4.6 is to learn how to draw representative graphs
of functions (i.e., graphs that display all of the significant features of a function: where it
is increasing or decreasing, any extrema, asymptotes and two features we’ll introduce
in section 4.5: concavity and inflection points). We draw each graph in a particular
viewing window (i.e., a particular range of x- and y-values). In the case of computer- or
calculator-generated graphs, the window is often chosen by the machine. To uncover
when significant features are hidden outside of a given window or to determine the
precise locations of features that we can see in a given window, we need some calculus.
EXAMPLE 4.1 Drawing a Graph
y
2
3
Draw a graph of f(x) = 2x + 9x − 24x − 10 showing all local extrema.
10
Solution Many graphing calculators use the default window defined by
−10 ≤ x ≤ 10 and −10 ≤ y ≤ 10. Using this window, the graph of y = f(x) looks like
x that displayed in Figure 4.43, although the three segments shown are not
-10 10
particularly revealing. Instead of blindly manipulating the window in the hope that
a reasonable graph will magically appear, we stop briefly to determine where the
-10
function is increasing and decreasing. We have
Copyright © McGraw-Hill Education
2
2
FIGURE 4.43 f (x) = 6x + 18x − 24 = 6(x + 3x − 4)
3
2
y = 2x + 9x − 24x − 10 = 6(x − 1)(x + 4).

262 | Lesson 4-4 | Increasing and Decreasing Functions