Page 10 - mobile Workbook-chapter 2

2.5 Particle size

Municipal refuse is possibly the worst imaginable material for particle size analysis, and yet

much of the MSW processing technology depends on an accurate description of particle size.

The size distribution of solid waste components is important for improving the rate of chemical

reactions; in other words, smaller particle sizes provide greater surface area and thus more rapid
reaction with microorganisms in a compost pile, or more rapid combustion in an incinerator. Size

distribution is also an important consideration in the recovery of materials, for example, with the

use of processing equipment such as a trommel screen or a magnetic separator.

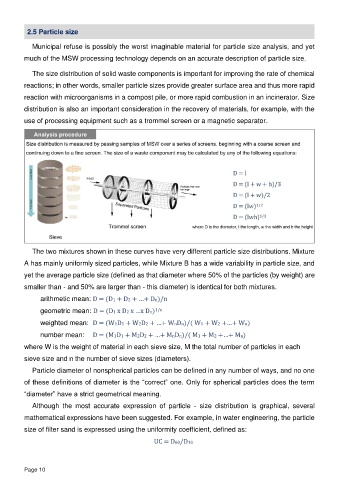
The two mixtures shown in these curves have very different particle size distributions. Mixture

A has mainly uniformly sized particles, while Mixture B has a wide variability in particle size, and

yet the average particle size (defined as that diameter where 50% of the particles (by weight) are
smaller than - and 50% are larger than - this diameter) is identical for both mixtures.

arithmetic mean: D = (D1 + D2 + …+ Dn)/n

geometric mean: D = (D1 x D2 x …x Dn) 1/n
weighted mean: D = (W1D1 + W2D2 + …+ WnDn)/( W1 + W2 +…+ Wn)

number mean: D = (M1D1 + M2D2 + …+ MnDn)/( M1 + M2 +…+ Mn)
where W is the weight of material in each sieve size, M the total number of particles in each

sieve size and n the number of sieve sizes (diameters).
Particle diameter of nonspherical particles can be defined in any number of ways, and no one

of these definitions of diameter is the “correct” one. Only for spherical particles does the term

“diameter” have a strict geometrical meaning.
Although the most accurate expression of particle - size distribution is graphical, several

mathematical expressions have been suggested. For example, in water engineering, the particle
size of filter sand is expressed using the uniformity coefficient, defined as:

UC = D60/D10

Page 10