Page 176 - Mathematics Coursebook

18.4 Calculating the volume of cuboids

18.4 Calculating the volume of cuboids

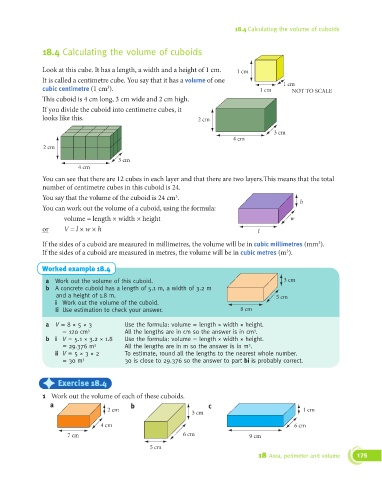
Look at this cube. It has a length, a width and a height of 1 cm. 1 cm
It is called a centimetre cube. You say that it has a volume of one
cubic centimetre (1 cm ). 1 cm 1 cm
3
NOT TO SCALE
2 cm

!is cuboid is 4 cm long, 3 cm wide and 2 cm high.
If you divide the cuboid into centimetre cubes, it
3 cm
4 cm
looks like this. 2 cm
3 cm
4 cm
2 cm

3 cm
4 cm
2 cm
You can see that there are 12 cubes in each layer and that there are two layers.!is means that the total

number of centimetre cubes in this cuboid is 24. 4 cm 3 cm
You say that the volume of the cuboid is 24 cm . h
3
You can work out the volume of a cuboid, using the formula:
volume = length × width × height w

or V = l × w × h l

If the sides of a cuboid are measured in millimetres, the volume will be in cubic millimetres (mm ).
3
If the sides of a cuboid are measured in metres, the volume will be in cubic metres (m ).
3
Worked example 18.4

a Work out the volume of this cuboid. 3 cm
b A concrete cuboid has a length of 5.1 m, a width of 3.2 m
and a height of 1.8 m. 5 cm
i Work out the volume of the cuboid.
Ii Use estimation to check your answer. 8 cm

a V = 8 × 5 × 3 Use the formula: volume = length × width × height.
= 120 cm All the lengths are in cm so the answer is in cm .
3
3
b i V = 5.1 × 3.2 × 1.8 Use the formula: volume = length × width × height.
= 29.376 m All the lengths are in m so the answer is in m .
3
3
ii V = 5 × 3 × 2 To estimate, round all the lengths to the nearest whole number.
= 30 m 30 is close to 29.376 so the answer to part bi is probably correct.
3
) Exercise 18.4

1 Work out the volume of each of these cuboids.
a b c
2 cm 1 cm
3 cm
4 cm 6 cm
7 cm 6 cm 9 cm

5 cm
18 Area, perimeter and volume 175