Page 179 - Mathematics Coursebook

18.5 Calculating the surface area of cubes and cuboids

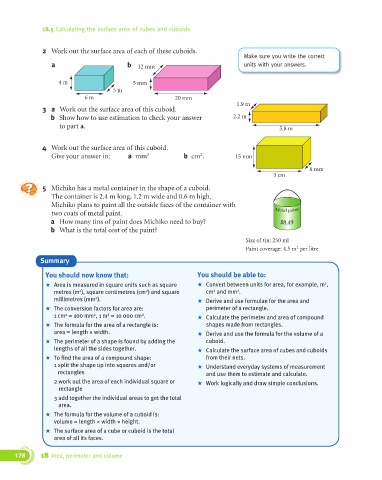
2 Work out the surface area of each of these cuboids.
Make sure you write the correct
a b 12 mm units with your answers.

4 m 5 mm
5 m
6 m 20 mm
1.9 m
3 a Work out the surface area of this cuboid.
b Show how to use estimation to check your answer 2.2 m
to part a. 5.8 m

4 Work out the surface area of this cuboid.
Give your answer in: a mm b cm . 15 mm
2
2
8 mm
3 cm
5 Michiko has a metal container in the shape of a cuboid.
The container is 2.4 m long, 1.2 m wide and 0.6 m high.
Michiko plans to paint all the outside faces of the container with Metal paint
two coats of metal paint.
a How many tins of paint does Michiko need to buy? $8.49
b What is the total cost of the paint?
Size of tin: 250 ml
Paint coverage: 4.5 m per litre
2
Summary

You should now know that: You should be able to:
2
+ Area is measured in square units such as square + Convert between units for area, for example, m ,
2
2
metres (m ), square centimetres (cm ) and square cm and mm .
2
2
millimetres (mm ). + Derive and use formulae for the area and
2
+ The conversion factors for area are: perimeter of a rectangle.
2
2
2
1 cm = 100 mm , 1 m = 10 000 cm . + Calculate the perimeter and area of compound
2
+ The formula for the area of a rectangle is: shapes made from rectangles.
area = length × width. + Derive and use the formula for the volume of a
+ The perimeter of a shape is found by adding the cuboid.
lengths of all the sides together. + Calculate the surface area of cubes and cuboids

+ To find the area of a compound shape: from their nets.
1 split the shape up into squares and/or + Understand everyday systems of measurement
rectangles and use them to estimate and calculate.
2 work out the area of each individual square or + Work logically and draw simple conclusions.
rectangle
3 add together the individual areas to get the total
area.
+ The formula for the volume of a cuboid is:
volume = length × width × height.
+ The surface area of a cube or cuboid is the total
area of all its faces.

178 18 Area, perimeter and volume