Page 183 - Mathematics Coursebook

19.1 Interpreting and drawing pictograms, bar charts, bar-line graphs and frequency diagrams

A bar-line graph is similar to a bar chart, but you use thick lines instead of bars.

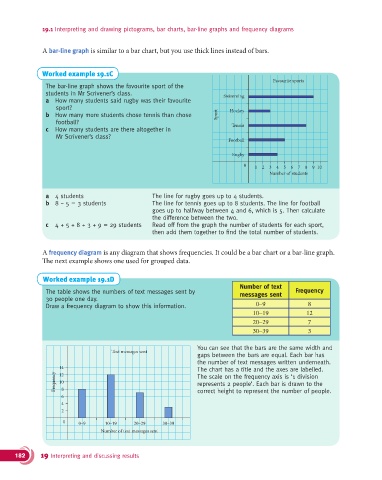
Worked example 19.1C
Favourite sports
The bar-line graph shows the favourite sport of the
students in Mr Scrivener’s class. Swimming
a How many students said rugby was their favourite
sport?
b How many more students chose tennis than chose Sport Hockey
football?
c How many students are there altogether in Tennis
Mr Scrivener’s class?
Football

Rugby
0 1 2 3 4 5 6 7 8 9 10
Number of students

a 4 students The line for rugby goes up to 4 students.
b 8 − 5 = 3 students The line for tennis goes up to 8 students. The line for football
goes up to halfway between 4 and 6, which is 5. Then calculate
the difference between the two.
c 4 + 5 + 8 + 3 + 9 = 29 students Read off from the graph the number of students for each sport,
then add them together to find the total number of students.

A frequency diagram is any diagram that shows frequencies. It could be a bar chart or a bar-line graph.
#e next example shows one used for grouped data.

Worked example 19.1D
Number of text
The table shows the numbers of text messages sent by messages sent Frequency
30 people one day.
Draw a frequency diagram to show this information. 0–9 8
10–19 12
20–29 7
30–39 3

You can see that the bars are the same width and
Text messages sent
gaps between the bars are equal. Each bar has
the number of text messages written underneath.
14 The chart has a title and the axes are labelled.
Frequency 12 8 The scale on the frequency axis is ‘1 division
10
represents 2 people’. Each bar is drawn to the
6 correct height to represent the number of people.
4
2
0 0–9 10–19 20–29 30–39
Number of text messages sent

182 19 Interpreting and discussing results