Page 11 - Module 1 in MATH 1 (Calculus)
P. 11
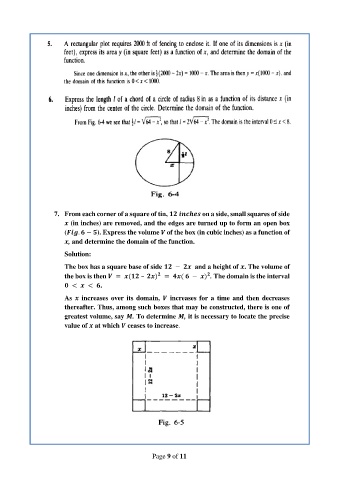
7. From each corner of a square of tin, on a side, small squares of side
(in inches) are removed, and the edges are turned up to form an open box
( . − ). Express the volume of the box (in cubic inches) as a function of
, and determine the domain of the function.
Solution:
The box has a square base of side − and a height of . The volume of
the box is then = ( – ) = ( − ) . The domain is the interval
< < .
As increases over its domain, increases for a time and then decreases
thereafter. Thus, among such boxes that may be constructed, there is one of
greatest volume, say . To determine , it is necessary to locate the precise
value of at which ceases to increase.
Page 9 of 11