Page 59 - Instrumentation and Measurement

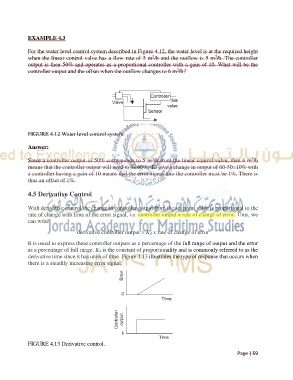
EXAMPLE 4.3

For the water level control system described in Figure 4.12, the water level is at the required height
when the linear control valve has a flow rate of 5 m3/h and the outflow is 5 m3/h. The controller
output is then 50% and operates as a proportional controller with a gain of 10. What will be the
controller output and the offset when the outflow changes to 6 m3/h?

FIGURE 4.12 Water level control system.

Answer:
Since a controller output of 50% corresponds to 5 m3/h from the linear control valve, then 6 m3/h
means that the controller output will need to be 60%. To give a change in output of 60-50=10% with
a controller having a gain of 10 means that the error signal into the controller must be 1%. There is
thus an offset of 1%.

4.5 Derivative Control

With derivative control the change in controller output from the set point value is proportional to the
rate of change with time of the error signal, i.e. controller output α rate of change of error. Thus, we
can write

derivative controller output = Kd x rate of change of error
It is usual to express these controller outputs as a percentage of the full range of output and the error
as a percentage of full range. Kd is the constant of proportionality and is commonly referred to as the
derivative time since it has units of time. Figure 4.13 illustrates the type of response that occurs when
there is a steadily increasing error signal.

FIGURE 4.13 Derivative control.

Page | 59