Page 14 - FINAL CFA I SLIDES JUNE 2019 DAY 4

Session Unit 3:

12. Hypothesis Testing
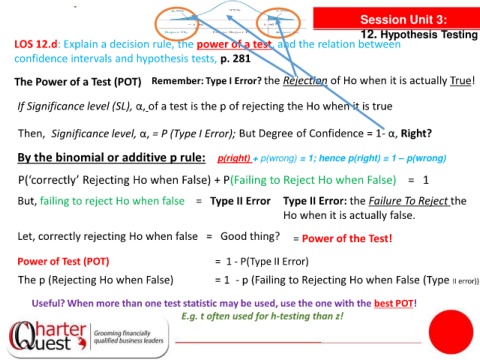
LOS 12.d: Explain a decision rule, the power of a test, and the relation between

confidence intervals and hypothesis tests, p. 281

The Power of a Test (POT) Remember: Type I Error? the Rejection of Ho when it is actually True!

If Significance level (SL), α, of a test is the p of rejecting the Ho when it is true

Then, Significance level, α, = P (Type I Error); But Degree of Confidence = 1- α, Right?

By the binomial or additive p rule: p(right) + p(wrong) = 1; hence p(right) = 1 – p(wrong)

P(‘correctly’ Rejecting Ho when False) + P(Failing to Reject Ho when False) = 1

But, failing to reject Ho when false = Type II Error Type II Error: the Failure To Reject the
Ho when it is actually false.

Let, correctly rejecting Ho when false = Good thing? = Power of the Test!

Power of Test (POT) = 1 - P(Type II Error)

The p (Rejecting Ho when False) = 1 - p (Failing to Rejecting Ho when False (Type II error)).

Useful? When more than one test statistic may be used, use the one with the best POT!
E.g. t often used for h-testing than z!