Page 39 - FINAL CFA I SLIDES JUNE 2019 DAY 3
P. 39
LOS 10.e: Define a Discrete Uniform Random Session Unit 3:
Variable, a Bernoulli random variable, and a 10. Common Probability Distributions
binomial random variable, p.217
LOS 10.f: Calculate and interpret probabilities given the discrete uniform and the binomial distribution functions
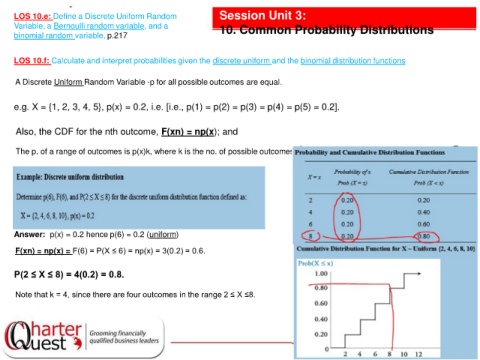
A Discrete Uniform Random Variable -p for all possible outcomes are equal.
e.g. X = {1, 2, 3, 4, 5}, p(x) = 0.2, i.e. [i.e., p(1) = p(2) = p(3) = p(4) = p(5) = 0.2].
Also, the CDF for the nth outcome, F(xn) = np(x); and
The p. of a range of outcomes is p(x)k, where k is the no. of possible outcomes in the range.
Answer: p(x) = 0.2 hence p(6) = 0.2 (uniform)
F(xn) = np(x) = F(6) = P(X ≤ 6) = np(x) = 3(0.2) = 0.6.
P(2 ≤ X ≤ 8) = 4(0.2) = 0.8.
Note that k = 4, since there are four outcomes in the range 2 ≤ X ≤8.