Page 41 - FINAL CFA I SLIDES JUNE 2019 DAY 3

LOS 10.e: Define a Discrete Uniform Random Session Unit 3:
Variable, a Bernoulli random variable, and a binomial
random variable. 10. Common Probability Distributions

LOS 10.f: Calculate and interpret probabilities given the discrete uniform and the binomial DF

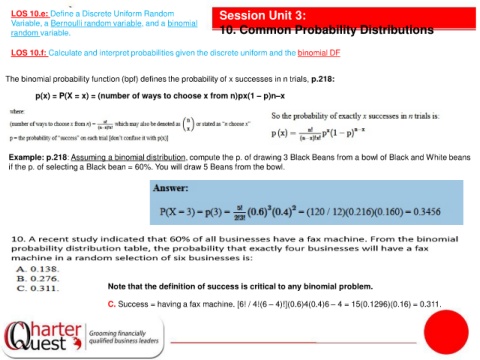
The binomial probability function (bpf) defines the probability of x successes in n trials, p.218:

p(x) = P(X = x) = (number of ways to choose x from n)px(1 – p)n–x

Example: p.218: Assuming a binomial distribution, compute the p. of drawing 3 Black Beans from a bowl of Black and White beans
if the p. of selecting a Black bean = 60%. You will draw 5 Beans from the bowl.

Note that the definition of success is critical to any binomial problem.

C. Success = having a fax machine. [6! / 4!(6 – 4)!](0.6)4(0.4)6 – 4 = 15(0.1296)(0.16) = 0.311.