Page 88 - Microsoft Word - 00 CIMA F1 Prelims STUDENT 2018.docx

Chapter 8

The tabular approach

When data in the exam is given in tabular form and there is no indication about the
demand function, and/or when there is no simple linear relationship between output
and profit – the tabular approach is likely to be the best to define optimum profit and
the associated selling price.

$ $ $ $ $ $

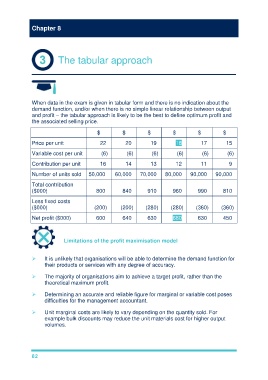
Price per unit 22 20 19 18 17 15

Variable cost per unit (6) (6) (6) (6) (6) (6)
Contribution per unit 16 14 13 12 11 9

Number of units sold 50,000 60,000 70,000 80,000 90,000 90,000

Total contribution
($000) 800 840 910 960 990 810

Less fixed costs
($000) (200) (200) (280) (280) (360) (360)

Net profit ($000) 600 640 630 680 630 450

Limitations of the profit maximisation model

 It is unlikely that organisations will be able to determine the demand function for
their products or services with any degree of accuracy.

 The majority of organisations aim to achieve a target profit, rather than the
theoretical maximum profit.

 Determining an accurate and reliable figure for marginal or variable cost poses
difficulties for the management accountant.

 Unit marginal costs are likely to vary depending on the quantity sold. For
example bulk discounts may reduce the unit materials cost for higher output
volumes.

82