Page 86 - Microsoft Word - 00 CIMA F1 Prelims STUDENT 2018.docx

Chapter 8

Procedure for establishing the maximum price of a product

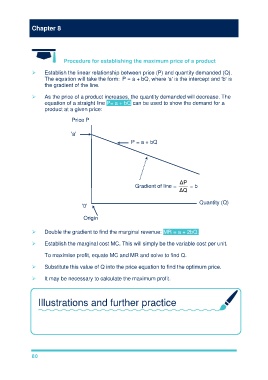
 Establish the linear relationship between price (P) and quantity demanded (Q).
The equation will take the form: P = a + bQ, where 'a' is the intercept and 'b' is
the gradient of the line.

 As the price of a product increases, the quantity demanded will decrease. The
equation of a straight line P= a + bQ can be used to show the demand for a
product at a given price:

Price P

'a'

P = a + bQ

Gradient of line = = b

Quantity (Q)
'0'

Origin

 Double the gradient to find the marginal revenue: MR = a + 2bQ.

 Establish the marginal cost MC. This will simply be the variable cost per unit.

To maximise profit, equate MC and MR and solve to find Q.

 Substitute this value of Q into the price equation to find the optimum price.

 It may be necessary to calculate the maximum profit.

Illustrations and further practice

Now read the illustration ‘The MR = MC diagram’ from Chapter 8 and attempt
examples 1 to 4.

80