Page 6 - TRIAL 2

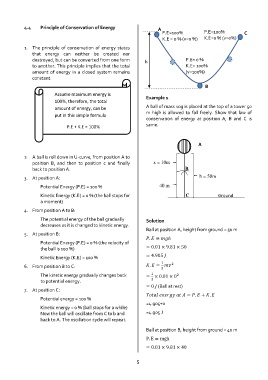
4.4 Principle of Conservation of Energy A
P.E=100% P.E=100% C

K.E = 0 % (v=0 %) K.E=0 % (v=0%)

1. The principle of conservation of energy states
that energy can neither be created nor
destroyed, but can be converted from one form h P.E= 0 %

to another. This principle implies that the total K.E= 100%
amount of energy in a closed system remains (v=100%)
constant.

B
Assume maximum energy is Example 1
100%, therefore, the total

P.E+K.E=100% be
amount of energy, can A ball of mass 10g is placed at the top of a tower 50
m high is allowed to fall freely. Show that law of
put in this simple formula
conservation of energy at position A, B and C is

P.E + K.E = 100% same.

A

2. A ball is roll down in U-curve, from position A to
position B, and then to position c and finally x = 10m
back to position A. B
h = 50m
3. At position A:
Potential Energy (P.E) = 100 % 40 m

Kinetic Energy (K.E) = 0 % (the ball stops for C Ground
a moment)

4. From position A to B:
The potential energy of the ball gradually Solution
decreases as it is changed to kinetic energy.
Ball at position A, height from ground = 50 m
5. At position B:
. = ℎ
Potential Energy (P.E) = 0 % (the velocity of
the ball is 100 %) = 0.01 × 9.81 × 50
= 4.905
Kinetic Energy (K.E) = 100 %
1 2
6. From position B to C: . =
2
1
The kinetic energy gradually changes back = × 0.01 × 0
2
to potential energy. 2
= 0 (Ball at rest)
7. At position C:
= . + .
Potential energy = 100 %
=4.905+0
Kinetic energy = 0 % (ball stops for a while)
Now the ball will oscillate from C to b and =4.905 J
back to A. The oscillation cycle will repeat.
Ball at position B, height from ground = 40 m

P. E = mgh
= 0.01 × 9.81 × 40

5