Page 7 - TRIAL 2

= 3.924 J
B
Total energy at A = Total energy at B , therefore

(Total energy at B) 4.905 = P. E + K. E
B
B
4.905 = 3.924 + K. E x
B
K. E = 4.905 − 3.924
B
= 0.981 J A
Total energy at B = P. E + K. E

=3.924+0.981 Solution
=4.905 J
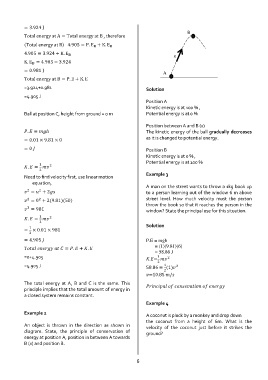
Position A

Kinetic energy is at 100 % ,
Ball at position C, height from ground = 0 m Potential energy is at 0 %

Position between A and B (x)
. = ℎ The kinetic energy of the ball gradually decreases
as it is changed to potential energy.
= 0.01 × 9.81 × 0

= 0 Position B
Kinetic energy is at 0 % ,
Potential energy is at 100 %
1 2
. =
2
Example 3
Need to find velocity first, use linear motion
equation,
A man on the street wants to throw a 1kg book up
2
2
= + 2 to a person learning out of the window 6 m above
2
2
= 0 + 2(9.81)(50) street level. How much velocity must the person
throw the book so that it reaches the person in the
2
= 981 window? State the principal use for this situation.
1 2
. =
2
Solution
1
= × 0.01 × 981
2
= 4.905 P.E = mgh
= (1)(9.81)(6)
= . + .
= 58.86 J
=0+4.905 1 2
.=
2
1
=4.905 J 58.86 = (1)
2
2
=10.85 /

The total energy at A, B and C is the same. This
principle implies that the total amount of energy in
a closed system remains constant.
Example 4

Example 2 A coconut is pluck by a monkey and drop down
the coconut from a height of 6m. What is the
An object is thrown in the direction as shown in velocity of the coconut just before it strikes the
diagram. State, the principle of conservation of ground?
energy at position A, position in between A towards

B (x) and position B.

6