Page 113 - Cambridge+Checkpoint+Mathematics+Coursebook+9

12.1 Tessellating shapes

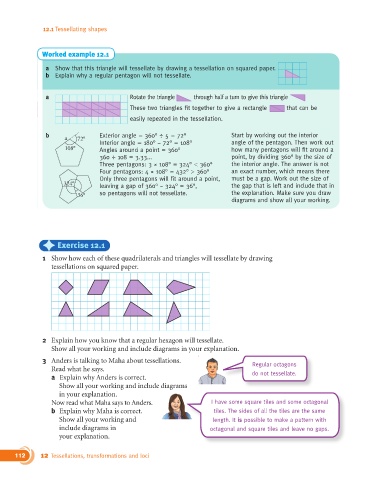
Worked example 12.1

a Show that this triangle will tessellate by drawing a tessellation on squared paper.
b Explain why a regular pentagon will not tessellate.

a Rotate the triangle through half a turn to give this triangle

These two triangles fi t together to give a rectangle that can be
easily repeated in the tessellation.

b a 72° Exterior angle = 360° ÷ 5 = 72° Start by working out the interior
Interior angle = 180° − 72° = 108° angle of the pentagon. Then work out
324°
108° Angles around a point = 360° how many pentagons will fi t around a
360 ÷ 108 = 3.33... point, by dividing 360° by the size of
36°
Three pentagons: 3 × 108° = 324° < 360° the interior angle. The answer is not
Four pentagons: 4 × 108° = 432° > 360° an exact number, which means there
a 72° Only three pentagons will fi t around a point, must be a gap. Work out the size of
108° 324° leaving a gap of 360° − 324° = 36°, the gap that is left and include that in
36° so pentagons will not tessellate. the explanation. Make sure you draw
diagrams and show all your working.

) Exercise 12.1

1 Show how each of these quadrilaterals and triangles will tessellate by drawing
tessellations on squared paper.

2 Explain how you know that a regular hexagon will tessellate.
Show all your working and include diagrams in your explanation.
3 Anders is talking to Maha about tessellations. Regular octagons
Read what he says. do not tessellate.
a Explain why Anders is correct.
Show all your working and include diagrams
in your explanation.
Now read what Maha says to Anders. I have some square tiles and some octagonal
b Explain why Maha is correct. tiles. The sides of all the tiles are the same
Show all your working and length. It is possible to make a pattern with
include diagrams in octagonal and square tiles and leave no gaps.
your explanation.

112 12 Tessellations, transformations and loci