Page 117 - Cambridge+Checkpoint+Mathematics+Coursebook+9

12.3 Transforming shapes

12.3 Transforming shapes

You can use a combination of re#ections, translations and rotations to transform a shape.

You can also describe the transformation that maps an object onto its image.

5P EFTDSJCF B SFĘFDUJPO ZPV NVTU HJWF t UIF FRVBUJPO PG UIF NJSSPS MJOF
5P EFTDSJCF B USBOTMBUJPO ZPV NVTU HJWF t UIF DPMVNO WFDUPS
5P EFTDSJCF B SPUBUJPO ZPV NVTU HJWF t UIF DFOUSF PG SPUBUJPO
t UIF OVNCFS PG EFHSFFT PG UIF SPUBUJPO PS GSBDUJPO PG B
whole turn)
t UIF EJSFDUJPO PG UIF SPUBUJPO DMPDLXJTF PS BOUJDMPDLXJTF

Note that when a rotation is 180° (half a turn) you do not need to give the
direction of the rotation as the image of the object will be the same whether you
rotate it clockwise or anticlockwise.

Worked example 12.3

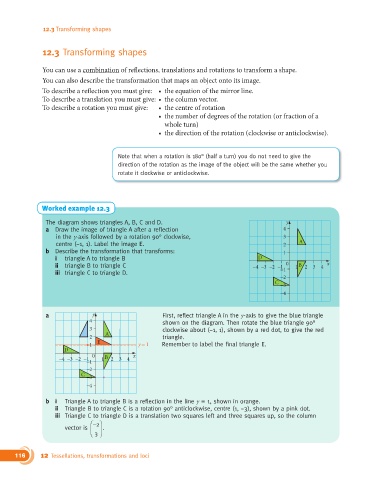
The diagram shows triangles A, B, C and D. y
a Draw the image of triangle A after a refl ection 4
in the y-axis followed by a rotation 90° clockwise, 3
centre (−1, 1). Label the image E. 2 A
b Describe the transformation that transforms: 1
i triangle A to triangle B D
ii triangle B to triangle C –4 –3 –2 –1 0 1 B 2 3 4 x
iii triangle C to triangle D. –1
–2
C
–3
–4

a y First, refl ect triangle A in the y-axis to give the blue triangle
4 shown on the diagram. Then rotate the blue triangle 90°
3 clockwise about (−1, 1), shown by a red dot, to give the red
A
2 triangle.
E
1 y = 1 Remember to label the fi nal triangle E.
D
0 B x
–4 –3 –2 –1 1 2 3 4
–1
–2
C
–3
–4

b i Triangle A to triangle B is a refl ection in the line y = 1, shown in orange.
ii Triangle B to triangle C is a rotation 90° anticlockwise, centre (1, −3), shown by a pink dot.
iii Triangle C to triangle D is a translation two squares left and three squares up, so the column
−  2 
vector is   .
 3 

116 12 Tessellations, transformations and loci