Page 72 - Cambridge+Checkpoint+Mathematics+Coursebook+9

7.4 Dividing fractions

2 Work out these divisions.
Write each answer in its lowest terms and as a mixed number when appropriate.
a 3 ÷ 5 b 7 ÷ 2 c 11 ÷ 3 d 4 ÷ 2 e 8 ÷ 4 f 7 ÷ 3
4 7 9 5 12 5 5 3 9 7 8 4
5
g 6 ÷ 3 h 5 ÷ 15 i 25 ÷ j 6 ÷ 9 k 8 ÷ 12 l 9 ÷ 15
7 14 6 24 32 8 7 10 15 25 28 42
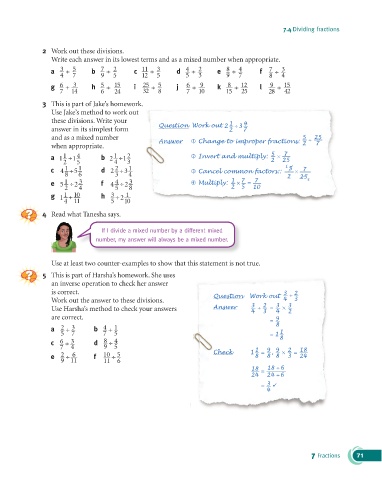
3 This is part of Jake’s homework.
Use Jake’s method to work out
these divisions. Write your Question Work out 2 1 4
answer in its simplest form 2 ÷ 3 7
and as a mixed number Answer c Change to improper fractions: 5 ÷ 25
when appropriate. 2 7
1
4
1
a 1 ÷ 1 b 2 ÷ 1 2 d Invert and multiply: 5 × 7
2 5 4 3 2 25
2
1
1
c 4 ÷ 5 d 2 ÷ 3 1 e Cancel common factors:: 1 5 × 7
8 6 3 4 2 25
3
4
1
e 5 ÷ 2 f 4 ÷ 2 3 f Multiply: 1 × 7 = 7 5
2 4 5 8 2 5 10
1 10
g 1 ÷ h 3 ÷ 2 1
4 11 5 10
4 Read what Tanesha says.
If I divide a mixed number by a different mixed
number, my answer will always be a mixed number.

Use at least two counter-examples to show that this statement is not true.
5 This is part of Harsha’s homework. She uses
an inverse operation to check her answer
is correct. Question Work out 3 ÷ 2
Work out the answer to these divisions. 4 3
Use Harsha’s method to check your answers Answer 3 ÷ 2 = 3 × 3
3
4
4
2
are correct. 9
= 8
3
a 2 ÷ b 4 ÷ 1 1
5 7 7 5 = 1 8
3
c 6 ÷ d 8 ÷ 4
7 4 9 5 1 9 9 2 18
Check
,
e 2 ÷ 6 f 10 ÷ 5 Check 1 8 = 8 8 × 3 = 24
9 11
11
6
18 18 ÷ 6
24 = 24 ÷ 6
= 3 9
4

7 Fractions 71