Page 111 - Maxwell House

NEOCLASSICAL THEORY OF INTERACTION 91

where the dimensionless parameter n = √ is

called the index of refraction. At first glance no
worries while and are positive values that

guarantees the positiveness of refraction index.
However, in 1968 Russian scientist Viktor
Veselago asked and answered the question [14],
“What we can expect if < 0 and < 0

simultaneously and the refraction coefficient
becomes negative n =−�| |?” Figure 2.8.2
19

demonstrates the surprising refraction effect
following from Snell’s law. The left-side image is Figure 2.8.2 Straw image
the conventional view of a straw in the glass filled
with normal water (n = 1.3). Meanwhile, the right-side image is the appearance of the same
straw in the same glass but the ordinary water is replaced by “water” with a fictional refractive
index n =−1.3. The
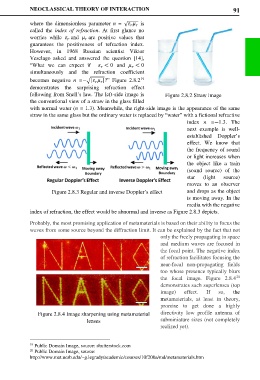
next example is well-
established Doppler’s
effect. We know that
the frequency of sound
or light increases when
the object like a train
(sound source) of the
star (light source)
moves to an observer
Figure 2.8.3 Regular and inverse Doppler’s effect and drops as the object
is moving away. In the
media with the negative
index of refraction, the effect would be abnormal and inverse as Figure 2.8.3 depicts.
Probably, the most promising application of metamaterials is based on their ability to focus the
waves from some source beyond the diffraction limit. It can be explained by the fact that not
only the freely propagating in space
and medium waves are focused in
the focal point. The negative index
of refraction facilitates focusing the
near-focal non-propagating fields
too whose presence typically blurs
the focal image. Figure 2.8.4
20
demonstrates such superlenses (top
image) effect. If so, the
metamaterials, at least in theory,
promise to get done a highly
Figure 2.8.4 Image sharpening using metamaterial directivity low profile antenna of
lenses subminiature sizes (not completely
realized yet).

19 Public Domain Image, source: shutterstock.com
20 Public Domain Image, source:
http://www.mat.ucsb.edu/~g.legrady/academic/courses/10f200a/md/metamaterials.htm