Page 129 - Maxwell House

NEOCLASSICAL THEORY OF INTERACTION 109

If so,

Φ ()
1 = −ℰ () (2.118)
1

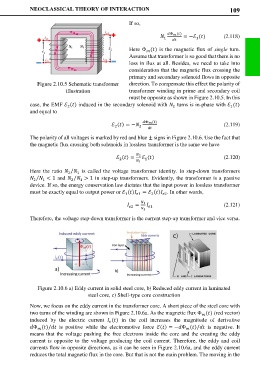
Here Φ () is the magnetic flux of single turn.

Assume that transformer is so good that there is no
loss in flux at all. Besides, we need to take into
consideration that the magnetic flux crossing the
primary and secondary solenoid flows in opposite
Figure 2.10.5 Schematic transformer direction. To compensate this effect the polarity of
illustration transformer winding in prime and secondary coil
must be opposite as shown in Figure 2.10.5. In this
case, the EMF ℰ () induced in the secondary solenoid with turns is in-phase with ℰ ()
2 2 1
and equal to
Φ ()
ℰ () = − (2.119)
2 2

The polarity of all voltages is marked by red and blue ± signs in Figure 2.10.6. Use the fact that
the magnetic flux crossing both solenoids in lossless transformer is the same we have

2
ℰ () = ℰ () (2.120)
1
2
1
Here the ratio is called the voltage transformer identity. In step-down transformers
⁄
2
1
< 1 and > 1 in step-up transformers. Evidently, the transformer is a passive
⁄
⁄
2
1
2
1
device. If so, the energy conservation law dictates that the input power in lossless transformer
must be exactly equal to output power or ℰ () 1 = ℰ () . In other words,
1
2
2
1
2 = (2.121)
1
2
Therefore, the voltage step-down transformer is the current step-up transformer and vice versa.

Figure 2.10.6 a) Eddy current in solid steel core, b) Reduced eddy current in laminated
steel core, c) Shell-type core construction
Now, we focus on the eddy current in the transformer core. A short piece of the steel core with
two turns of the winding are shown in Figure 2.10.6a. As the magnetic flux Φ () (red vector)

induced by the electric current () in the coil increases the magnitude of derivative

Φ ()/ is positive while the electromotive force ℰ() = −Φ ()/ is negative. It

means that the voltage pushing the free electrons inside the core and the creating the eddy
current is opposite to the voltage producing the coil current. Therefore, the eddy and coil
currents flow in opposite directions, as it can be seen in Figure 2.10.6a, and the eddy current
reduces the total magnetic flux in the core. But that is not the main problem. The moving in the