Page 161 - Maxwell House

POYNTING's THEOREM 141

The doughnut-shape and cylindrical ring cavity shown in Figure 3.2.1a are exemplary. From
the above discussion surrounding the uniqueness theorem in loss-free space-frequency domain
follows that any Maxwell’s equation solution with the given sources and non-zero boundary
conditions always
should be supplemented
with the infinite
spectrum of resonance
solutions corresponding
t = T/8 t = T/4 t = 3T/8
to the zero boundary
conditions (3.67). If

t = 0 t = T/2 there is no macroscopic
loss in a medium, the
field oscillations are not
damped. Without the
t = 7T/8 t = 3T/4 t = 5T/8 initial conditions likes
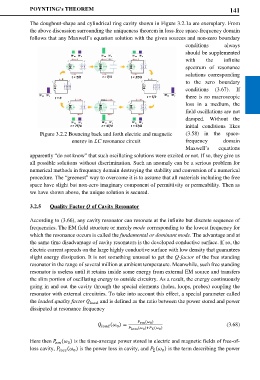
Figure 3.2.2 Bouncing back and forth electric and magnetic (3.58) in the space-
energy in ℒC resonance circuit frequency domain
Maxwell’s equations
apparently “do not know” that such oscillating solutions were excited or not. If so, they give us
all possible solutions without discrimination. Such an anomaly can be a serious problem for
numerical methods in frequency domain destroying the stability and conversion of a numerical
procedure. The “greenest” way to overcome it is to assume that all materials including the free
space have slight but non-zero imaginary component of permittivity or permeability. Then as
we have shown above, the unique solution is secured.

3.2.5 Quality Factor Q of Cavity Resonator

According to (3.66), any cavity resonator can resonate at the infinite but discrete sequence of
frequencies. The EM field structure or merely mode corresponding to the lowest frequency for
which the resonance occurs is called the fundamental or dominant mode. The advantage and at
the same time disadvantage of cavity resonators is the developed conductive surface. If so, the
electric current spreads on the large highly conductive surface with low density that guarantees
slight energy dissipation. It is not something unusual to get the Q-factor of the free standing
resonator in the range of several million at ambient temperature. Meanwhile, such free standing
resonator is useless until it retains inside some energy from external EM source and transfers
the slim portion of oscillating energy to outside circuitry. As a result, the energy continuously
going in and out the cavity through the special elements (holes, loops, probes) coupling the
resonator with external circuitries. To take into account this effect, a special parameter called

the loaded quality factor and is defined as the ratio between the power stored and power
dissipated at resonance frequency

( 0 )
( ) = (3.68)
0
( 0 )+ Σ ( 0 )
Here then ( ) is the time-average power stored in electric and magnetic fields of free-of-

0
loss cavity, ( ) is the power loss in cavity, and ( ) is the term describing the power
0 Σ 0