Page 164 - Maxwell House

144 Chapter 3

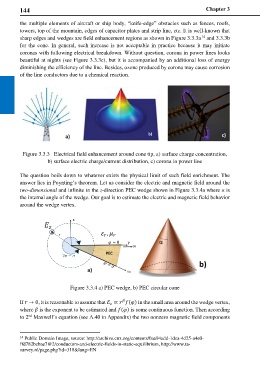
the multiple elements of aircraft or ship body, “knife-edge” obstacles such as fences, roofs,
towers, top of the mountain, edges of capacitor plates and strip line, etc. It is well-known that
14
sharp edges and wedges are field enhancement regions as shown in Figure 3.3.3a and 3.3.3b
for the cone. In general, such increase is not acceptable in practice because it may initiate
coronas with following electrical breakdown. Without question, corona in power lines looks
beautiful at nights (see Figure 3.3.3c), but it is accompanied by an additional loss of energy
diminishing the efficiency of the line. Besides, ozone produced by corona may cause corrosion
of the line conductors due to a chemical reaction.

Figure 3.3.3 Electrical field enhancement around cone tip, a) surface charge concentration,
b) surface electric charge/current distribution, c) corona in power line

The question boils down to whatever exists the physical limit of such field enrichment. The
answer lies in Poynting’s theorem. Let us consider the electric and magnetic field around the
two-dimensional and infinite in the z-direction PEC wedge shown in Figure 3.3.4a where α is
the internal angle of the wedge. Our goal is to estimate the electric and magnetic field behavior
around the wedge vertex.

Figure 3.3.4 a) PEC wedge, b) PEC circular cone

If → 0, it is reasonable to assume that ∝ () in the small area around the wedge vertex,

where is the exponent to be estimated and () is some continuous function. Then according
to 2 Maxwell’s equation (see A.40 in Appendix) the two nonzero magnetic field components
nd

14 Public Domain Image, source: http://archive.cnx.org/contents/0aa94add-1dca-4d25-a4e0-
ffd782bcbae7@2/conductors-and-electric-fields-in-static-equilibrium, http://www.ta-
survey.nl/page.php?id=318&lang=EN