Page 167 - Maxwell House

POYNTING's THEOREM 147

radii and < are connected by a thin copper wire. We assume that the spheres are so far
2
1
1
apart that the charge distribution on each of the spheres practically undisturbed and very close
to uniform. As well, we will neglect the influence of charges accumulated on the thin wire.
What is the ratio of the charges / residing on the surfaces of the spheres and what is their
1
2
surface density ratio 1 / 2 ? When the connection between the spheres is established, the
1 1
sphere with higher potential = will be partly discharged through the wire while
1
4 0 1
1 2
charging the other sphere with the potential 2 = . At the end of this process, both
4 0 2
spheres must be at the same electric potential or = (if they were not, Coulomb’s forces
1 2
push charges until the equilibrium is reached). In other words, the equipotential condition
implies
1 1 1 2
= (3.80)
4 0 1 4 0 2
2
Therefore, = where 1,2 = 1,2 4 . We can then rewrite the charge ratio as
⁄
⁄
1,2
2
1
2
1
follows
⁄
2 = 1 ( ) (3.81)
1
2
and 2 → ∞ as the radius of surface curvature → 0. It may seem surprising at first that the
2
sphere carrying lesser charge has the higher charge density. But recall that the charge in
2
(3.79) decreases proportional while the small sphere surface shrinks much faster as .
2
2
2
Consequently, according to (3.77) the E-field and surface charge density is at their most intense
just where the radius of conductive surface curvature is the smallest. In general, in areas of high
local changes of E-field the alternative magnetic field manifests its most intense too since H-
field is the curl or space differential of E-field. Moreover, recall that according to the boundary
conditions in Table 2.2 the current density is equal to tangential component of H-field on

the PEC surfaces. Therefore, we can
expect that in areas where the radius
of conductive surface curvature is the
smallest the electric current surface
density is highest.
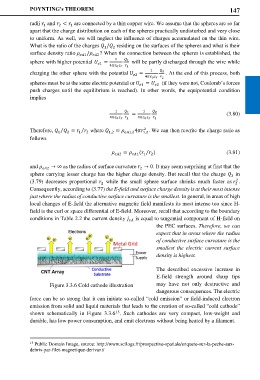
The described excessive increase in
E-field strength around sharp tips
Figure 3.3.6 Cold cathode illustration may have not only destructive and
dangerous consequences. The electric
force can be so strong that it can initiate so-called “cold emission” or field-induced electron
emission from solid and liquid materials that leads to the creation of so-called “cold cathode”
15
shown schematically in Figure 3.3.6 . Such cathodes are very compact, low-weight and
durable, has low power consumption, and emit electrons without being heated by a filament.

15 Public Domain Image, source: http://www.scilogs.fr/prospective-spatiale/enquete-sur-la-peche-aux-
debris-par-filet-magnetique-derivant/