Page 171 - Maxwell House
P. 171
POYNTING's THEOREM 151
18
structures as close to each other as possible . Then the only difference between the incident,
reflected, and aperture field vectors are their intensities (amplitude and phase). If so, we can
omit the vector symbols and write
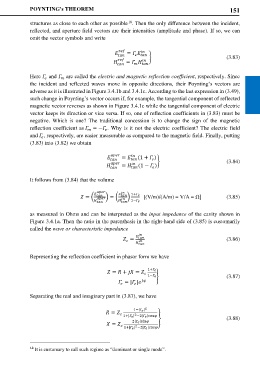
=
� (3.83)
=
Here and are called the electric and magnetic reflection coefficient, respectively. Since
the incident and reflected waves move in opposite directions, their Poynting’s vectors are
adverse as it is illustrated in Figure 3.4.1b and 3.4.1c. According to the last expression in (3.49),
such change in Poynting’s vector occurs if, for example, the tangential component of reflected
magnetic vector reverses as shown in Figure 3.4.1c while the tangential component of electric
vector keeps its direction or vice versa. If so, one of reflection coefficients in (3.83) must be
negative. Which is one? The traditional concession is to change the sign of the magnetic
reflection coefficient as = − . Why is it not the electric coefficient? The electric field
and , respectively, are easier measurable as compared to the magnetic field. Finally, putting
(3.83) into (3.82) we obtain
= (1 + )
� (3.84)
= (1 − )
It follows from (3.84) that the volume
1+
= � � = � � [(V/m)/(A/m) = V/A = Ω] (3.85)
1−
as measured in Ohms and can be interpreted as the input impedance of the cavity shown in
Figure 3.4.1a. Then the ratio in the parenthesis in the right-hand side of (3.85) is customarily
called the wave or characteristic impedance
(3.86)
=
Representing the reflection coefficient in phasor form we have
1+
= + =
1− � (3.87)
= | |
Separating the real and imaginary part in (3.87), we have
1−| | 2
=
1+| | 2 −2| |cos � (3.88)
2| |sin
=
1+| | 2 −2| |cos
18 It is customary to call such regime as “dominant or single mode”.