Page 170 - Maxwell House

150 Chapter 3

operate using such traditional values as voltage, current, inductance, etc. Evidently, that is not
quite right as we move to higher frequencies up to optical spectrum and behind. We discussed
this subject in previous chapters (see Section 2.2.8 in Chapter 2) and found that most of the
traditionally lumped circuit conceptions including voltage cannot be defined uniquely in the
case of AC and RF circuits. Let us demonstrate how to bypass this difficulty introducing the
concept of the reflection coefficient. It is worth to point out that the reflection coefficient is a
measurement-friendly parameter.

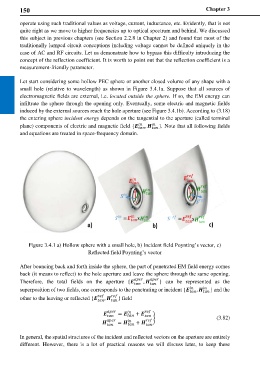
Let start considering some hollow PEC sphere or another closed volume of any shape with a
small hole (relative to wavelength) as shown in Figure 3.4.1a. Suppose that all sources of
electromagnetic fields are external, i.e. located outside the sphere. If so, the EM energy can
infiltrate the sphere through the opening only. Eventually, some electric and magnetic fields
induced by the external sources reach the hole aperture (see Figure 3.4.1b). According to (3.18)
the entering sphere incident energy depends on the tangential to the aperture (called terminal
plane) components of electric and magnetic field { , }. Note that all following fields

and equations are treated in space-frequency domain.

Figure 3.4.1 a) Hollow sphere with a small hole, b) Incident field Poynting’s vector, c)
Reflected field Poynting’s vector

After bouncing back and forth inside the sphere, the part of penetrated EM field energy comes
back (it means to reflect) to the hole aperture and leave the sphere through the same opening.

Therefore, the total fields on the aperture { , } can be represented as the

superposition of two fields, one corresponds to the penetrating or incident { , } and the

other to the leaving or reflected { , } field

= +

� (3.82)
= +

In general, the spatial structures of the incident and reflected vectors on the aperture are entirely
different. However, there is a lot of practical reasons we will discuss later, to keep these