Page 172 - Maxwell House

152 Chapter 3

Note that in any passive (there are no field sources) system the reflected wave magnitude cannot
exceed the incident one meaning that 0 ≤ | | ≤ 1. It is a remarkable fact though that (3.88)

defines the unique impedance for any given or measured value of reflection coefficient.

However, the final picture is not so rosy because we defined the impedances in (3.85) and (3.86)
as the ratio of the transverse electric and the transverse magnetic field. Unluckily, in some cases,
these ratios are not constants and are the functions of the transverse spatial coordinates. There
are several ways to fix this problem, and we are going to discuss them later. The simplest and
most reasonable technique is to normalize all values to a 1-Ohm introducing the normalized
impedance as

⁄
= = + = (1 + ) (1 − )⁄ ⎫

2
1−| | ⎪
= 1+| | 2 −2| |cos (3.89)

⎬
2| |sin
= 1+| | 2 −2| |cos ⎪

⎭
The equities in (3.89) give us a one-to-one relationship between the reflection coefficient ,

the real part (resistance) and imaginary part (reactance) of normalized impedance .

This fact was used by American engineer Phillip H. Smith who proposed in 1939 a sophisticated
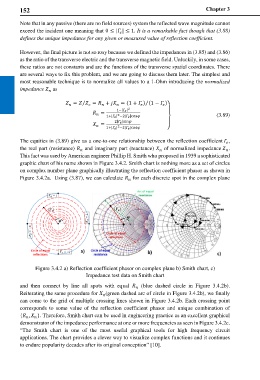
graphic chart of his name shown in Figure 3.4.2. Smith chart is nothing more as a set of circles
on complex number plane graphically illustrating the reflection coefficient phasor as shown in
Figure 3.4.2a. Using (3.87), we can calculate for each discrete spot in the complex plane

Figure 3.4.2 a) Reflection coefficient phasor on complex plane b) Smith chart, c)
Impedance test data on Smith chart
and then connect by line all spots with equal (blue dashed circle in Figure 3.4.2b).

Reiterating the same procedure for (green dashed arc of circle in Figure 3.4.2b), we finally

can come to the grid of multiple crossing lines shown in Figure 3.4.2b. Each crossing point
corresponds to some value of the reflection coefficient phasor and unique combination of
{ , }. Therefore, Smith chart can be used in engineering practice as an excellent graphical

demonstrator of the impedance performance at one or more frequencies as seen in Figure 3.4.2c.
“The Smith chart is one of the most useful graphical tools for high frequency circuit
applications. The chart provides a clever way to visualize complex functions and it continues
to endure popularity decades after its original conception” [10].