Page 55 - Maxwell House

BASIC EQUATIONS OF MACROSCOPIC ELECTRODYNAMICS 35

point in the direction of the magnetic field vector H. Because of the angular symmetry the
magnitude |H| is constant along this circle L and can be taken out of the integral in (1.66).
Therefore,

2� || = (1.67)

If so, we can define the magnetic field strength as a numerical value of current when the

circumference is 1m long.
Applying Stokes’s theorem (see Appendix) to (1.65) we can transform the linear integral on the
left-hand side of (1.65) as
∮ ∘ = ∬ x ∘ (1.68)

The closed contour L and surface area A are the same as shown in Figure 1.6.8. Combining
(1.65) and (1.68) and collecting all terms we obtain
0
∬ � x − − � ∘ = 0 (1.69)

q ∘ is the only portion of total electric current

qv - Here = ∬

that leaves or enters the volume V through the surface area A.
Pronouncing our “magic” words about arbitrary surface area A we
finally get 2 Maxwell’s equation in differential form
nd
0 x = + (1.70)

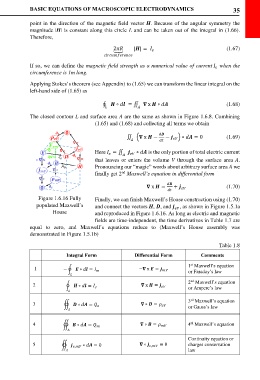
Figure 1.6.16 Fully Finally, we can finish Maxwell’s House construction using (1.70)
populated Maxwell’s and connect the vectors H, , and , as shown in Figure 1.5.1a

House and reproduced in Figure 1.6.16. As long as electric and magnetic
fields are time-independent, the time derivatives in Table 1.7 are
equal to zero, and Maxwell’s equations reduce to (Maxwell’s House assembly was
demonstrated in Figure 1.5.1b)
Table 1.8

Integral Form Differential Form Comments
st
1 Maxwell’s equation
1 − � ∘ = − x = or Faraday’s law

2 Maxwell’s equation
nd
2 � ∘ = x = or Ampere’s law

rd
3 � ∘ = ∘ = 3 Maxwell’s equation
or Gauss’s law

th
4 � ∘ = ∘ = 4 Maxwell’s equation

Continuity equation or
5 � , ∘ = 0 ∘ , = 0 charges conservation
law