Page 50 - Maxwell House

30 Chapter 1

− x − = (1.45)

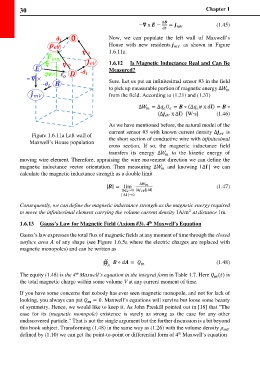
0 Now, we can populate the left wall of Maxwell’s
House with new residents as shown in Figure
1.6.11a.
q 1.6.12 Is Magnetic Inductance Real and Can Be
qv - Measured?
Sure. Let us put an infinitesimal sensor #3 in the field
to pick up measurable portion of magnetic energy ∆

from the field. According to (1.21) and (1.37)

∆ = ∆ = ∘ (∆ x ) = ∘

(∆ x ∆) [W⋅s] (1.46)

As we have mentioned before, the natural model of the
current sensor #3 with known current density ∆ is
Figure 1.6.11a Left wall of
Maxwell’s House population the short section of conductive wire with infinitesimal
cross section. If so, the magnetic inductance field
transfers its energy ∆ to the kinetic energy of

moving wire element. Therefore, appraising the wire movement direction we can define the
magnetic inductance vector orientation. Then measuring ∆ and knowing | ∆ | we can

calculate the magnetic inductance strength as a double limit
∆
|| = lim (1.47)
Δ →0 |∆ ||∆|
| ∆ |→0
Consequently, we can define the magnetic inductance strength as the magnetic energy required
to move the infinitesimal element carrying the volume current density 1A/m at distance 1m.
2
1.6.13 Gauss’s Law for Magnetic Field (Axiom #3). 4 Maxwell’s Equation
th
Gauss’s law expresses the total flux of magnetic fields at any moment of time through the closed
surface area of any shape (see Figure 1.6.5a where the electric charges are replaced with
magnetic monopoles) and can be written as

∯ ∘ = (1.48)

The equity (1.48) is the 4 Maxwell’s equation in the integral form in Table 1.7. Here () is
th

the total magnetic charge within some volume V at any current moment of time.
If you have some concerns that nobody has ever seen magnetic monopole, and not for lack of
looking, you always can put = 0. Maxwell’s equations will survive but loose some beauty

of symmetry. Hence, we would like to keep it. As John Preskill pointed out in [18] that “The
case for its (magnetic monopole) existence is surely as strong as the case for any other
undiscovered particle.” That is not the single argument but the further discussion is a bit beyond

this book subject. Transforming (1.48) in the same way as (1.26) with the volume density
defined by (1.10) we can get the point-to-point or differential form of 4 Maxwell’s equation
th