Page 148 - Mechatronics with Experiments

134 MECHATRONICS
N 1
T 1 r 1

1

N 2
r 2

T 2
2

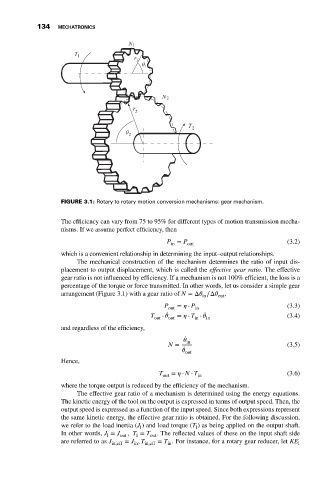
FIGURE 3.1: Rotary to rotary motion conversion mechanisms: gear mechanism.

The efficiency can vary from 75 to 95% for different types of motion transmission mecha-
nisms. If we assume perfect efficiency, then
P = P (3.2)
in out
which is a convenient relationship in determining the input–output relationships.
The mechanical construction of the mechanism determines the ratio of input dis-
placement to output displacement, which is called the effective gear ratio. The effective
gear ratio is not influenced by efficiency. If a mechanism is not 100% efficient, the loss is a
percentage of the torque or force transmitted. In other words, let us consider a simple gear
arrangement (Figure 3.1) with a gear ratio of N =Δ ∕Δ out ,
in
P out = ⋅ P in (3.3)
T out ⋅ ̇ out = ⋅ T ⋅ ̇ in (3.4)
in
and regardless of the efficiency,

̇ in
N = (3.5)
̇ out
Hence,
T out = ⋅ N ⋅ T in (3.6)
where the torque output is reduced by the efficiency of the mechanism.
The effective gear ratio of a mechanism is determined using the energy equations.
The kinetic energy of the tool on the output is expressed in terms of output speed. Then, the
output speed is expressed as a function of the input speed. Since both expressions represent
the same kinetic energy, the effective gear ratio is obtained. For the following discussion,
we refer to the load inertia (J ) and load torque (T ) as being applied on the output shaft.
l
l
In other words, J = J out , T = T out . The reflected values of these on the input shaft side
l
l
are referred to as J in,eff = J , T in,eff = T . For instance, for a rotary gear reducer, let KE l
in
in