Page 153 - Mechatronics with Experiments

MECHANISMS FOR MOTION TRANSMISSION 139
The mass moment of inertia of the cylindrical load,
1 2
J = ⋅ m ⋅ (d∕2) (3.42)
l
2
1 2 2
= ⋅ ⋅ ⋅ (d∕2) ⋅ l ⋅ (d∕2) (3.43)
2
1 4
= ⋅ ⋅ ⋅ l ⋅ (d∕2) (3.44)
2
1 4 2
= ⋅ (0.286∕386) ⋅ ⋅ 2.0 ⋅ (3∕2) lb ⋅ in ⋅ s (3.45)
2
2
= 0.0118 lb ⋅ in ⋅ s (3.46)
2
where g = 386 in∕s , the gravitational acceleration, is used to convert the weight density
to mass density. Hence, the reflected inertia and torque are
1 −3 2
J in,eff = ⋅ 0.0118 = 0.118 × 10 lb ⋅ in ⋅ s (3.47)
10 2
1
T in,eff = ⋅ 200 = 20.0lb ⋅ in (3.48)
10

3.3 ROTARY TO TRANSLATIONAL MOTION
TRANSMISSION MECHANISMS

The rotary to translational motion transmission mechanisms convert rotary motion to linear
translational motion. Translational motion is also refered as linear motion. Both terms will
be used interchangeably in the following discussions. In addition, torque input is converted
to force at the output. It should be noted that all of the rotary to translational motion
transmission mechanisms discussed here are back drivable, meaning that they also make
the conversion in the reverse direction.

3.3.1 Lead-Screw and Ball-Screw Mechanisms
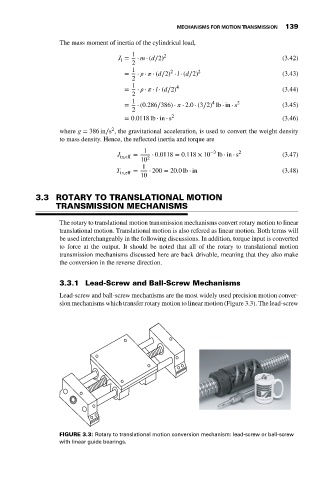
Lead-screw and ball-screw mechanisms are the most widely used precision motion conver-
sion mechanisms which transfer rotary motion to linear motion (Figure 3.3). The lead-screw

FIGURE 3.3: Rotary to translational motion conversion mechanism: lead-screw or ball-screw
with linear guide bearings.