Page 158 - Mechatronics with Experiments

144 MECHATRONICS

Coupler
Rocker
arm
A
L
L A
Crank 2 R
L 3
θ 2
L 1
θ
θ B R 3
1
L 4
B L

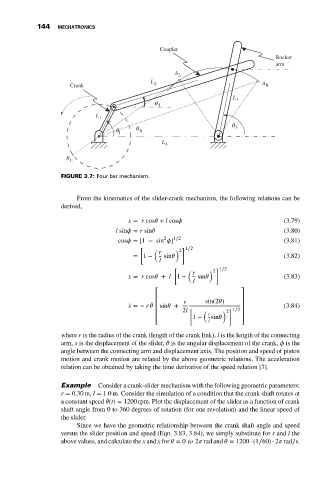
FIGURE 3.7: Four bar mechanism.

From the kinematics of the slider-crank mechanism, the following relations can be
derived,
x = r cos + l cos (3.79)
l sin = r sin (3.80)
2
cos = [1 − sin ] 1∕2 (3.81)
[ ] 1∕2
( r ) 2
= 1 − sin (3.82)
l
[ ] 1∕2
( r ) 2
x = r cos + l 1 − sin (3.83)
l
⎡ ⎤
⎢ ⎥
r sin(2 )
̇ x =− r sin + ⎥ (3.84)
̇ ⎢
⎢ 2l [ ( ) 2 ] 1∕2 ⎥
r
⎢ 1 − sin ⎥
⎣ l ⎦
where r is the radius of the crank (length of the crank link), l is the length of the connecting
arm, x is the displacement of the slider, is the angular displacement of the crank, is the
angle between the connecting arm and displacement axis. The position and speed of piston
motion and crank motion are related by the above geometric relations. The acceleration
relation can be obtained by taking the time derivative of the speed relation [7].

Example Consider a crank-slider mechanism with the following geometric parameters:
r = 0.30 m, l = 1.0 m. Consider the simulation of a condition that the crank shaft rotates at
̇
a constant speed (t) = 1200 rpm. Plot the displacement of the slider as a function of crank
shaft angle from 0 to 360 degrees of rotation (for one revolution) and the linear speed of
the slider.
Since we have the geometric relationship between the crank shaft angle and speed
versus the slider position and speed (Eqn. 3.83, 3.84), we simply substitute for r and l the
̇
above values, and calculate the x and ̇ x for = 0 to 2 rad and = 1200 ⋅ (1∕60) ⋅ 2 rad∕s.