Page 162 - Mechatronics with Experiments

148 MECHATRONICS
d x 2 Modified sine Sine
dθ 2 Modified trapezoidal

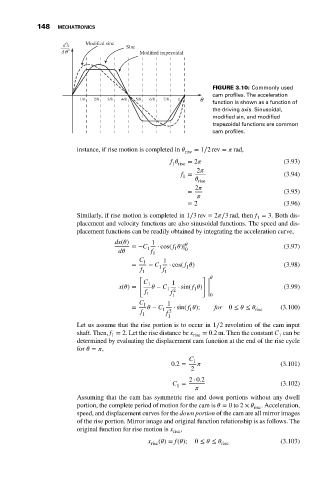
FIGURE 3.10: Commonly used
cam profiles. The acceleration
1/8 2/8 3/8 4/8 5/8 6/8 7/8 1 θ
function is shown as a function of
the driving axis. Sinusoidal,
modified sin, and modified
trapezoidal functions are common
cam profiles.

instance, if rise motion is completed in = 1∕2rev = rad,
rise
f = 2 (3.93)
1 rise
2
f = (3.94)
1

rise
2
= (3.95)

= 2 (3.96)
Similarly, if rise motion is completed in 1∕3rev = 2 ∕3 rad, then f = 3. Both dis-
1
placement and velocity functions are also sinusoidal functions. The speed and dis-
placement functions can be readily obtained by integrating the acceleration curve,
dx( ) 1
=−C 1 ⋅ cos( f )| (3.97)
1
d f 1 0
C 1 1
= − C 1 ⋅ cos( f ) (3.98)
1
f 1 f 1
[ ]
C 1 1 |
x( ) = − C 1 ⋅ sin( f ) | | (3.99)
1
f 1 f 1 2 | |0
C 1 1
= − C 1 ⋅ sin( f ); for 0 ≤ ≤ rise (3.100)
1
f 1 f 1 2
Let us assume that the rise portion is to occur in 1∕2 revolution of the cam input
shaft. Then, f = 2. Let the rise distance be x = 0.2 m. Then the constant C can be
1 rise 1
determined by evaluating the displacement cam function at the end of the rise cycle
for = ,
C 1
0.2 = (3.101)
2
2 ⋅ 0.2
C = (3.102)
1

Assuming that the cam has symmetric rise and down portions without any dwell
portion, the complete period of motion for the cam is = 0to2 × rise . Acceleration,
speed, and displacement curves for the down portion of the cam are all mirror images
of the rise portion. Mirror image and original function relationship is as follows. The
original function for rise motion is x rise ,
x rise ( ) = f( ); 0 ≤ ≤ rise (3.103)