Page 82 - Mechatronics with Experiments

68 MECHATRONICS

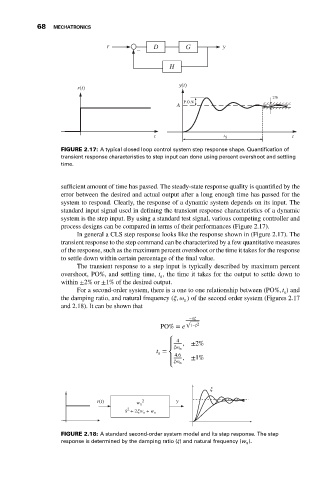
r _ D G y

H

y(t)
r(t)
2%
P.O.%
A

t t s t

FIGURE 2.17: A typical closed loop control system step response shape. Quantification of
transient response characteristics to step input can done using percent overshoot and settling
time.

sufficient amount of time has passed. The steady-state response quality is quantified by the
error between the desired and actual output after a long enough time has passed for the
system to respond. Clearly, the response of a dynamic system depends on its input. The
standard input signal used in defining the transient response characteristics of a dynamic
system is the step input. By using a standard test signal, various competing controller and
process designs can be compared in terms of their performances (Figure 2.17).
In general a CLS step response looks like the response shown in (Figure 2.17). The
transient response to the step command can be characterized by a few quantitative measures
of the response, such as the maximum percent overshoot or the time it takes for the response
to settle down within certain percentage of the final value.
The transient response to a step input is typically described by maximum percent
overshoot, PO%, and settling time, t , the time it takes for the output to settle down to
s
within ±2% or ±1% of the desired output.
For a second-order system, there is a one to one relationship between (PO%, t ) and
s
the damping ratio, and natural frequency ( , ) of the second order system (Figures 2.17
n
and 2.18). It can be shown that
−
√
PO%= e 1− 2
⎧ 4
, ±2%
⎪ n
t =
s
⎨
4.6
⎪ , ±1%
n
⎩
ξ
r(t) w n 2 y
2
s + 2 w + w n
ξ n
FIGURE 2.18: A standard second-order system model and its step response. The step
).
response is determined by the damping ratio ( ) and natural frequency (w n