Page 191 - Servo Motors and Industrial Control Theory -

188 Appendix B

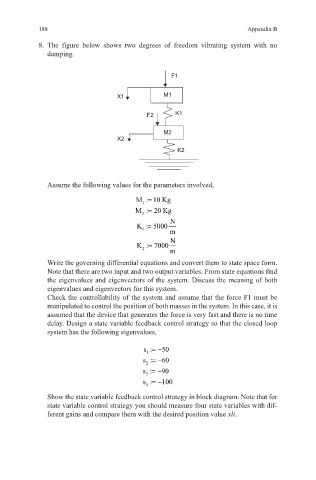
8. The figure below shows two degrees of freedom vibrating system with no
damping.

F1

X1 M1

F2 K1

M2
X2
K2

Assume the following values for the parameters involved,

=
M : 10 Kg
1
=
M : 20 Kg
2
N
=
K : 5000
1
m
N
=
K : 7000
2
m
Write the governing differential equations and convert them to state space form.
Note that there are two input and two output variables. From state equations find
the eigenvalues and eigenvectors of the system. Discuss the meaning of both
eigenvalues and eigenvectors for this system.
Check the controllability of the system and assume that the force F1 must be
manipulated to control the position of both masses in the system. In this case, it is
assumed that the device that generates the force is very fast and there is no time
delay. Design a state variable feedback control strategy so that the closed loop
system has the following eigenvalues,
s :=− 50
1
s :=− 60
2
s :=− 90
3
s :=− 100
4
Show the state variable feedback control strategy in block diagram. Note that for
state variable control strategy you should measure four state variables with dif-
ferent gains and compare them with the desired position value xli.