Page 195 - Servo Motors and Industrial Control Theory -

192 Appendix B

mass m to the cart, is negligible. Write the equations in state space form. You
should have four state variables. Determine the roots of the characteristic equa-
tions and show that the system is indeed unstable, for the following numerical
values of the parameters,
m = .5 kg
M = 5 kg
L = 0.6 m

Design state variable feedback control strategy so that all roots of the character-
istic equation move to the required position. Remember that before you design
state variable feedback you should check that indeed the system is controllable.
You decide this time where the roots should be located. The system must not
be too fast which require fast force variation, and they must not be too slow so
that the pendulum does not fall.
If you have any problems with this problem or require more information about
state space form refer to the book written by K. Ogata, third edition.
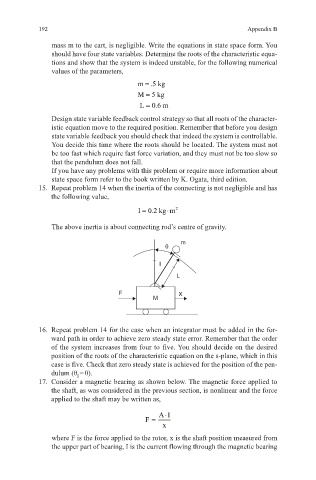
15. Repeat problem 14 when the inertia of the connecting is not negligible and has
the following value,

I = 0.2 kg m⋅ 2
The above inertia is about connecting rod’s centre of gravity.

m
θ

I
L

F X
M

16. Repeat problem 14 for the case when an integrator must be added in the for-
ward path in order to achieve zero steady state error. Remember that the order
of the system increases from four to five. You should decide on the desired
position of the roots of the characteristic equation on the s-plane, which in this
case is five. Check that zero steady state is achieved for the position of the pen-
dulum (θ = 0).
I
17. Consider a magnetic bearing as shown below. The magnetic force applied to
the shaft, as was considered in the previous section, is nonlinear and the force
applied to the shaft may be written as,

AI ⋅
F =
x
where F is the force applied to the rotor, x is the shaft position measured from
the upper part of bearing, I is the current flowing through the magnetic bearing