Page 63 - Buku Teks Digital Mate KSSM T5

BAB 2 Matriks
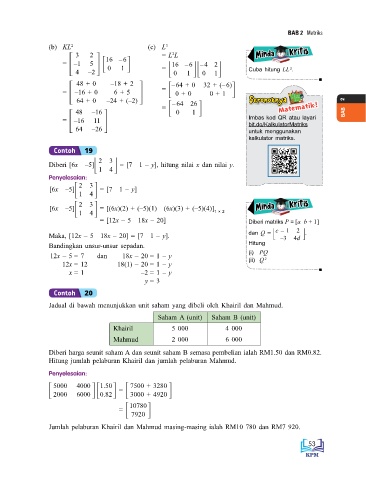
(b) KL 2 (c) L 3 2
3 3 2 16 – 6 = L L
3
= –1 5 4 0 1 4 3 16 – 6 43 – 4 2 4
2
4 –2 = 0 1 0 1 Cuba hitung LL .
3 48 + 0 –18 + 2 4 = 3 – 64 + 0 32 + (– 6) 4
= –16 + 0 6 + 5 0 + 0 0 + 1
64 + 0 –24 + (–2) – 64 26 Seronoknya 2
= 3 0 1 4 Matematik! BAB
–16
48
3 4 Imbas kod QR atau layari
= –16 11 bit.do/KalkulatorMatriks
64 –26 untuk menggunakan
kalkulator matriks.

Contoh 19

Diberi [6x –5] 3 2 3 4 = [7 1 – y], hitung nilai x dan nilai y.
4
1
Penyelesaian:
[6x –5] 3 2 3 4 = [7 1 – y]
1
4
[6x –5] 3 2 3 4 = [(6x)(2) + (–5)(1) (6x)(3) + (–5)(4)] 1 × 2
4
1

= [12x – 5 18x – 20] Diberi matriks P = [a b + 1]
Maka, [12x – 5 18x – 20] = [7 1 – y]. dan Q = 3 c – 1 2 4 .
–3 4d
Bandingkan unsur-unsur sepadan. Hitung
12x – 5 = 7 dan 18x – 20 = 1 – y (i) PQ
(ii) Q
2
12x = 12 18(1) – 20 = 1 – y
x = 1 –2 = 1 – y
y = 3
Contoh 20

Jadual di bawah menunjukkan unit saham yang dibeli oleh Khairil dan Mahmud.
Saham A (unit) Saham B (unit)
Khairil 5 000 4 000
Mahmud 2 000 6 000

Diberi harga seunit saham A dan seunit saham B semasa pembelian ialah RM1.50 dan RM0.82.
Hitung jumlah pelaburan Khairil dan jumlah pelaburan Mahmud.

Penyelesaian:
1.50
3 5000 4000 4 3 4 3 7500 + 3280 4
=
6000
2000
0.82
3000 + 4920
= 3 10780 4
7920
Jumlah pelaburan Khairil dan Mahmud masing-masing ialah RM10 780 dan RM7 920.

53
KPM