Page 4 - KI - Álgebra 3

Álgebra 3° Secundaria

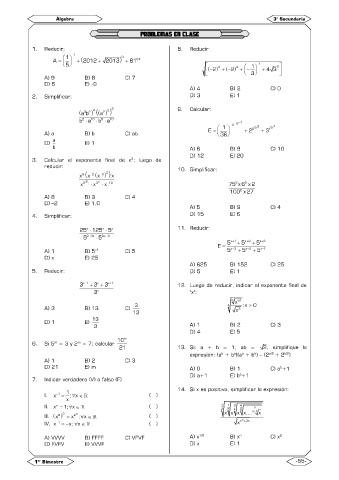
1. Reducir: 8. Reducir:
 1  1 0 1/4
A     2012  2013   81
 5  3 4  1  1 0 

   2    2    3   4 3  

A) 9 B) 8 C) 7
D) 6 E) -9
A) 4 B) 2 C) 0
2. Simplificar: D) 3 E) 1

4
2
3 2
 a
2
 a b     2 9. Calcular:

6
2
b  a 10  b  a 10  2  1
 1   4 0 5 3 1 5 7
A) a B) b C) ab E    36   2  3
a
D) E) 1
b A) 6 B) 8 C) 10
D) 12 E) 20
2
3. Calcular el exponente final de x ; luego de
reducir: 10. Simplificar:
2
x 6  x  2  x  3   x
2
3
x 2   x 3 2  x  10 75 x 6 x 2
2
100 x 27
2
A) 8 B) 3 C) 4
D) –2 E) 1,0
A) 5 B) 9 C) 4
4. Simplificar: D) 15 E) 6

25 125   x 5 x 11. Reducir:
x


5 2 3x  5 9x 3



E  5 x 1  5 x 2  5 x 3



–1
A) 1 B) 5 C) 5 5 x 3  5 x 2  5 x 1
D) x E) 25
A) 625 B) 152 C) 25
5. Reducir: D) 5 E) 1



3 x 1  3  x 3 x 1 12. Luego de reducir, indicar el exponente final de
3 x "x":
6 x 81
3 4 ;x  0
A) 3 B) 13 C) x 3
13
13
D) 1 E)
3 A) 1 B) 2 C) 3
D) 4 E) 5
10 m
m
6. Si 5 = 3 y 2 = 7; calcular
m
21 13. Si: a + b = 1; ab = 2, simplifique la
b/2
expresión: (a + b )(a + b ) – (2 a/2 + 2 )
b
a
a
b
A) 1 B) 2 C) 3
D) 21 E) m A) 0 B) 1 C) a +1
b
D) a+1 E) b +1
a
7. Indicar verdadero (V) o falso (F)

1 14. Si x es positivo, simplificar la expresión:

I. x  1  ; x  ( )
x
II. x  1; x  ( ) 2 1 2 1 3 4 4 5 n
o


5 5 x x x x.... n 1 x
III.  x 2   x ; x  ( )
2

2
IV. x  1   x; x  ( ) x n  3n


n
2
A) VVVV B) FFFF C) VFVF A) x 1/2 B) x C) x
D) FVFV E) VVVF D) x E) 1

er
1 Bimestre -55-