Page 62 - u4

P1: OSO/OVY
GO01962-Smith-v1.cls
UAE-Math-Grade-12-Vol-1-SE-718383-ch4
CHAPTER 4 • •
Applications of Differentiation
252 P2: OSO/OVY QC: OSO/OVY T1: OSO October 25, 2018 17:24 4-42
show two different shapes of decreasing functions. The one shown in Figure 4.55a is
concave up (slopes of tangent lines increasing) and the one shown in Figure 4.55b is
concave down (slopes of tangent lines decreasing). We summarize this in Definition 5.1.

DEFINITION 5.1
For a function f that is differentiable on an interval I, the graph of f is
′
(i) concave up on I if f is increasing on I.
′
(ii) concave down on I if f is decreasing on I.

′
Note that you can tell when f is increasing or decreasing from the derivative of f ′
′′
(i.e., f ). Theorem 5.1 connects concavity with what we already know about increasing
and decreasing functions. The proof is a straightforward application of Theorem 4.1 to
Definition 5.1.

THEOREM 5.1
′′
Suppose that f exists on an interval I.
′′
(i) If f (x) > 0 on I, then the graph of f is concave up on I.
′′
(ii) If f (x) < 0 on I, then the graph of f is concave down on I.

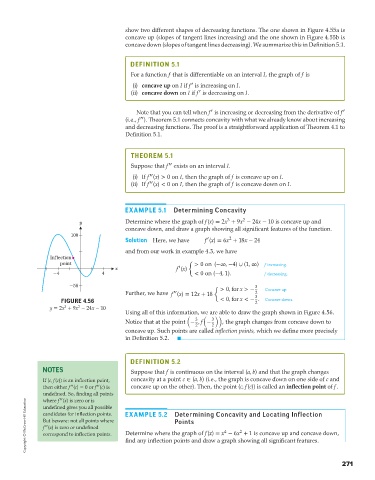
EXAMPLE 5.1 Determining Concavity
3
2
y Determine where the graph of f(x) = 2x + 9x − 24x − 10 is concave up and
concave down, and draw a graph showing all significant features of the function.
100
′
2
Solution Here, we have f (x) = 6x + 18x − 24
and from our work in example 4.3, we have
Inflection
point { > 0 on (−∞, −4) ∪ (1, ∞) f increasing.
′
x f (x)
-4 4 < 0on(−4, 1). f decreasing.
-50 { > 0, for x > − 3 Concave up.
′′
Further, we have f (x) = 12x + 18 2
3
FIGURE 4.56 < 0, for x < − . Concave down.
2
3
2
y = 2x + 9x − 24x − 10
Using all of this information, we are able to draw the graph shown in Figure 4.56.
( ( ))
3
Notice that at the point − ,f − 3 , the graph changes from concave down to
2 2
concave up. Such points are called inflection points, which we define more precisely
in Definition 5.2.
DEFINITION 5.2
NOTES Suppose that f is continuous on the interval (a, b) and that the graph changes
If (c, f(c)) is an inflection point, concavity at a point c ∈ (a, b) (i.e., the graph is concave down on one side of c and
′′
′′
then either f (c) = 0 or f (c) is concave up on the other). Then, the point (c, f(c)) is called an inflection point of f.
undefined. So, finding all points
′′
Copyright © McGraw-Hill Education candidates for inflection points. EXAMPLE 5.2 Determining Concavity and Locating Inflection
where f (x) is zero or is
undefined gives you all possible
But beware: not all points where
Points
′′
f (x) is zero or undefined
2
4
Determine where the graph of f(x) = x − 6x + 1 is concave up and concave down,
correspond to inflection points.
find any inflection points and draw a graph showing all significant features.
271