Page 66 - u4

P2: OSO/OVY
T1: OSO
P1: OSO/OVY
July 4, 2016
UAE_Math_Grade_12_Vol_1_SE_718383_ch3
GO01962-Smith-v1.cls
13:38
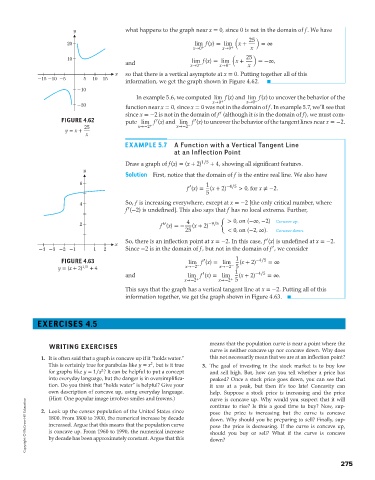
y QC: OSO/OVY what happens to the graph near x = 0, since 0 is not in the domain of f. We have
( 25 )
20 lim f(x) = lim x + =∞
x→0 + x→0 + x
( 25 )
10 lim f(x) = lim x + = −∞,
and x→0 − x→0 − x
x so that there is a vertical asymptote at x = 0. Putting together all of this
-15 -10 -5 5 10 15
information, we get the graph shown in Figure 4.62.
-10
In example 5.6, we computed lim f(x) and lim f(x) to uncover the behavior of the
−
+
x→0
x→0
-20 function near x = 0, since x = 0 was not in the domain of f. In example 5.7, we’ll see that
′
since x =−2 is not in the domain of f (although it is in the domain of f), we must com-
FIGURE 4.62 pute lim f (x) and lim f (x) to uncover the behavior of the tangent lines near x =−2.
′
′
y = x + 25 x→−2 + x→−2 −
x
EXAMPLE 5.7 A Function with a Vertical Tangent Line
at an Inflection Point
Draw a graph of f(x) = (x + 2) 1∕5 + 4, showing all significant features.
y
Solution First, notice that the domain of f is the entire real line. We also have
6 1
′
f (x) = (x + 2) −4∕5 > 0, for x ≠ −2.
5
4 So, f is increasing everywhere, except at x =−2 [the only critical number, where
′
f (−2) is undefined]. This also says that f has no local extrema. Further,
′′
2 f (x) =− 4 (x + 2) −9∕5 { > 0, on (−∞, −2) Concave up.
25 < 0, on (−2, ∞). Concave down.
′
So, there is an inflection point at x =−2. In this case, f (x) is undefined at x =−2.
x ′
-4 -3 -2 -1 1 2 Since −2 is in the domain of f, but not in the domain of f , we consider
′
FIGURE 4.63 lim f (x) = lim 1 (x + 2) −4∕5 =∞
−
y = (x + 2) 1∕5 + 4 x→−2 − x→−2 5
1
′
and lim f (x) = lim (x + 2) −4∕5 =∞.
+
x→−2 + x→−2 5
This says that the graph has a vertical tangent line at x =−2. Putting all of this
information together, we get the graph shown in Figure 4.63.

EXERCISES .5
4

WRITING EXERCISES means that the population curve is near a point where the
curve is neither concave up nor concave down. Why does
1. It is often said that a graph is concave up if it “holds water.” this not necessarily mean that we are at an inflection point?
2
This is certainly true for parabolas like y = x , but is it true 3. The goal of investing in the stock market is to buy low
2
for graphs like y = 1∕x ? It can be helpful to put a concept and sell high. But, how can you tell whether a price has
into everyday language, but the danger is in oversimplifica- peaked? Once a stock price goes down, you can see that
tion. Do you think that “holds water” is helpful? Give your it was at a peak, but then it’s too late! Concavity can
own description of concave up, using everyday language. help. Suppose a stock price is increasing and the price
(Hint: One popular image involves smiles and frowns.)
curve is concave up. Why would you suspect that it will
Copyright © McGraw-Hill Education 2. Look up the census population of the United States since pose the price is increasing but the curve is concave
continue to rise? Is this a good time to buy? Now, sup-
1800. From 1800 to 1900, the numerical increase by decade
down. Why should you be preparing to sell? Finally, sup-
increased. Argue that this means that the population curve
pose the price is decreasing. If the curve is concave up,
is concave up. From 1960 to 1990, the numerical increase
should you buy or sell? What if the curve is concave
by decade has been approximately constant. Argue that this
down?
275