Page 93 - u4

P1: OSO/OVY
GO01962-Smith-v1.cls
UAE_Math_Grade_12_Vol_1_SE_718383_ch4
QC: OSO/OVY
P2: OSO/OVY
P1: OSO/OVY
T1: OSO
GO01962-Smith-v1.cls
October 18, 2019
UAE_Math_Grade_12_Vol_1_SE_718383_ch4
19:54
CHAPTER 4 • •
Applications of Differentiation
282 P2: OSO/OVY QC: OSO/OVY T1: OSO October 18, 2019 19:54 4-72
282 CHAPTER 4 • • Applications of Differentiation 4-72
EXAMPLE 8.4 Estimating a Rate of Change in Economics
EXAMPLE 8.4 Estimating a Rate of Change in Economics
A small company estimates that when it spends x thousand dollars for advertising in
a year, its annual sales will be described by s = 60 − 40e −0.05x thousand dollars. The
A small company estimates that when it spends x thousand dollars for advertising in
four most recent annual advertising totals are given in the following table.
−0.05x
a year, its annual sales will be described by s = 60 − 40e
thousand dollars. The
Year advertising totals are given in the following table.
four most recent annual 1 2 3 4
Advertising Dollars 14,500 16,000 18,000 20,000
1
Year
2
3
4
16,000
18,000
20,000
′ 14,500
Advertising Dollars
Estimate the current (year 4) value of x (t) and the current rate of change of sales.
′
Estimate the current (year 4) value of x (t) and the current rate of change of sales.
Solution From the table, we see that the recent trend is for advertising to increase
′
by AED 2000 per year. A good estimate is then x (4) ≈ 2. Starting with the sales
Solution From the table, we see that the recent trend is for advertising to increase
equation ′
by AED 2000 per year. A good estimate is then x (4) ≈ 2. Starting with the sales
equation s(t) = 60 − 40e −0.05x(t) ,
we use the chain rule to obtain s(t) = 60 − 40e −0.05x(t) ,
′ to obtain
we use the chain rule
′
′
s (t) =−40e −0.05x(t) [−0.05x (t)] = 2x (t)e −0.05x(t) .
′
′
′
s (t) =−40e −0.05x(t) [−0.05x (t)] = 2x (t)e −0.05x(t) .
′
′
Using our estimate that x (4) ≈ 2 and since x(4) = 20, we get s (4) ≈ 2(2)e −1 ≈ 1.472.
′
′
Thus, sales are increasing at the rate of approximately AED 1472 per year. −1 ≈ 1.472.
Using our estimate that x (4) ≈ 2 and since x(4) = 20, we get s (4) ≈ 2(2)e
Thus, sales are increasing at the rate of approximately AED 1472 per year.
Notice that example 8.5 is similar to example 8.6 of section 2.8.
Notice that example 8.5 is similar to example 8.6 of section 2.8.
EXAMPLE 8.5 Tracking a Fast Jet
EXAMPLE 8.5 Tracking a Fast Jet
A spectator at an air show is trying to follow the flight of a jet. The jet follows a
straight path in front of the observer at 540 mph. At its closest approach, the jet
A spectator at an air show is trying to follow the flight of a jet. The jet follows a
passes 600 feet in front of the person. Find the maximum rate of change of the
straight path in front of the observer at 540 mph. At its closest approach, the jet
angle between the spectator’s line of sight and a line perpendicular to the flight
passes 600 feet in front of the person. Find the maximum rate of change of the
path, as the jet flies by.
angle between the spectator’s line of sight and a line perpendicular to the flight
path, as the jet flies by.
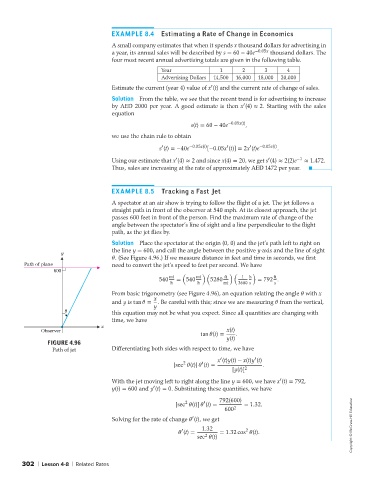
Solution Place the spectator at the origin (0, 0) and the jet’s path left to right on
the line y = 600, and call the angle between the positive y-axis and the line of sight
y Solution Place the spectator at the origin (0, 0) and the jet’s path left to right on
. (See Figure 4.96.) If we measure distance in feet and time in seconds, we first
the line y = 600, and call the angle between the positive y-axis and the line of sight
Path of plane y need to convert the jet’s speed to feet per second. We have
. (See Figure 4.96.) If we measure distance in feet and time in seconds, we first
600
Path of plane need to convert the jet’s speed to feet per second. We have
(
)(
)
)(
ft
600 540 mi = 540 mi 5280 ft 1 h = 792 .
s
h
h )(
(
h
ft
ft
1
540 mi = 540 mi 5280 mi )( 3600 s ) = 792 .
h
h
s
mi
3600 s
From basic trigonometry (see Figure 4.96), an equation relating the angle with x
and y is tan = x . Be careful with this; since we are measuring from the vertical, x
From basic trigonometry (see Figure 4.96), an equation relating the angle with
θ and y is tan = y x . Be careful with this; since we are measuring from the vertical,
this equation may not be what you expect. Since all quantities are changing with
y
θ time, we have
this equation may not be what you expect. Since all quantities are changing with
x time, we have
Observer x(t)
x tan (t) = .
y(t)
Observer x(t)
FIGURE 4.96 tan (t) = .
y(t)
Path of jet
FIGURE 4.96 Differentiating both sides with respect to time, we have
′ time, we have
′
Path of jet Differentiating both sides with respect to x (t)y(t) − x(t)y (t)
′
2
[sec (t)] (t) = x (t)y(t) − 2 ′ .
′
′
2
[sec (t)] (t) = [y(t)] x(t)y (t) .
2
[y(t)]
′
With the jet moving left to right along the line y = 600, we have x (t) = 792,
′
y(t) = 600 and y (t) = 0. Substituting these quantities, we have ′
With the jet moving left to right along the line y = 600, we have x (t) = 792,
′
y(t) = 600 and y (t) = 0. Substituting these quantities, we have
792(600)
′
2
[sec (t)] (t) = 792(600) = 1.32.
2
600
2
′
[sec (t)] (t) = = 1.32.
′
Solving for the rate of change (t), we get 600 2
′
1.32 get
Solving for the rate of change (t), we Copyright © McGraw-Hill Education
′
2
(t) = sec (t) = 1.32 cos (t).
2
′
2
(t) = 1.32 = 1.32 cos (t).
2
sec (t)
302 | Lesson 4-8 | Related Rates