Page 96 - u4

T1: OSO
P2: OSO/OVY
QC: OSO/OVY
P1: OSO/OVY
GO01962-Smith-v1.cls
UAE_Math_Grade_12_Vol_1_SE_718383_ch3
July 4, 2016
(b) Repeat for a sandpile for which the edge of the sandpile 13:38
forms an angle of 45 with the horizontal. EXPLORATORY EXERCISES
◦
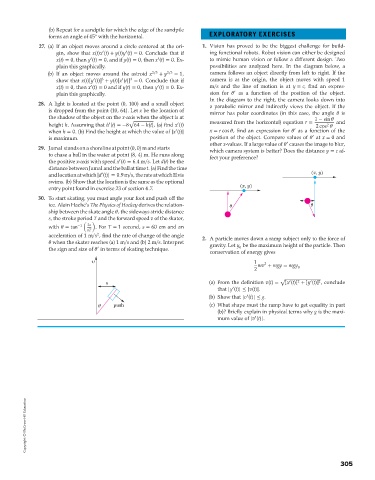
27. (a) If an object moves around a circle centered at the ori- 1. Vision has proved to be the biggest challenge for build-
′
′
gin, show that x(t)x (t) + y(t)y (t) = 0. Conclude that if ing functional robots. Robot vision can either be designed
′
′
x(t) = 0, then y (t) = 0, and if y(t) = 0, then x (t) = 0. Ex- to mimic human vision or follow a different design. Two
plain this graphically. possibilities are analyzed here. In the diagram below, a
(b) If an object moves around the astroid x 2∕3 + y 2∕3 = 1, camera follows an object directly from left to right. If the
′
3
′
3
show that x(t)[y (t)] + y(t)[x (t)] = 0. Conclude that if camera is at the origin, the object moves with speed 1
′
′
x(t) = 0, then x (t) = 0 and if y(t) = 0, then y (t) = 0. Ex- m/s and the line of motion is at y = c, find an expres-
′
plain this graphically. sion for as a function of the position of the object.
In the diagram to the right, the camera looks down into
28. A light is located at the point (0, 100) and a small object a parabolic mirror and indirectly views the object. If the
is dropped from the point (10, 64). Let x be the location of mirror has polar coordinates (in this case, the angle is
the shadow of the object on the x-axis when the object is at 1 − sin
√
′
′
height h. Assuming that h (t) =−8 64 − h(t), (a) find x (t) measured from the horizontal) equation r = 2 cos and
2
′
′ x = r cos , find an expression for as a function of the
when h = 0. (b) Find the height at which the value of |x (t)|
′
is maximum. position of the object. Compare values of at x = 0 and
other x-values. If a large value of causes the image to blur,
′
29. Jamal standsonashorelineatpoint(0,0)mandstarts which camera system is better? Does the distance y = c af-
to chase a ball in the water at point (8, 4) m. He runs along fect your preference?
′
the positive x-axis with speed x (t) = 6.4 m/s. Let d(t) be the
distancebetweenJamal andtheballattimet.(a)Findthetime
′
andlocationatwhich|d (t)| = 0.9m/s,therateatwhichElvis (x, y)
swims. (b) Show that the location is the same as the optional
entry point found in exercise 23 of section 4.7. (x, y)
30. To start skating, you must angle your foot and push off the
ice. Alain Haché’s The Physics of Hockey derives the relation- θ θ
ship between the skate angle , the sideways stride distance
s, the stroke period T and the forward speed v of the skater,
( )
with = tan −1 2s . For T = 1 second, s = 60 cm and an
vT
2
acceleration of 1 m/s , find the rate of change of the angle 2. A particle moves down a ramp subject only to the force of
when the skater reaches (a) 1 m/s and (b) 2 m/s. Interpret gravity. Let y be the maximum height of the particle. Then
′
the sign and size of in terms of skating technique. 0
conservation of energy gives
υ 1 mv + mgy = mgy
2
2 0
2
2
′
′
s (a) From the definition v(t) = √ [x (t)] + [y (t)] , conclude
′
that |y (t)| ≤ |v(t)|.
′
(b) Show that |v (t)| ≤ g.
θ push (c) What shape must the ramp have to get equality in part
(b)? Briefly explain in physical terms why g is the maxi-
′
mum value of |v (t)|.
4.9 RATES OF CHANGE IN ECONOMICS AND THE SCIENCES
It has often been said that mathematics is the language of nature. Today, the concepts of
calculus are being applied in virtually every field of human endeavor. The applications
in this section represent but a small sampling of some elementary uses of the derivative.
Copyright © McGraw-Hill Education can hardly pick up a newspaper without finding reference to some rates (e.g., inflation
Recall that the derivative of a function gives the instantaneous rate of change of
that function. So, when you see the word rate, you should be thinking derivative. You
rate, interest rate, etc.). These can be thought of as derivatives. There are also many
familiar quantities that you might not recognize as rates of change. Our first example,
which comes from economics, is of this type.
305