Page 95 - u4

P1: OSO/OVY
GO01962-Smith-v1.cls
UAE-Math-Grade-12-Vol-1-SE-718383-ch4
• •
CHAPTER 4
284 P2: OSO/OVY QC: OSO/OVY T1: OSO November 6, 2018 17:59 4-74
Applications of Differentiation
Year 0 1 2
Adver. 16,000 18,000 20,000
′
Estimate the value of x (2) and the current (year 2) rate of
change of sales.
18 ft
14. Suppose that the average yearly cost per item for producing
x items of a business product is C(x) = 12 + 94 . The three
x
most recent yearly production figures are given in the table. 6 ft
Year 0 1 2
Prod. (x) 8.2 8.8 9.4 x s
Exercise 19
′
Estimate the value of x (2) and the current (year 2) rate of
change of the average cost. 20. Boyle’s law for a gas at constant temperature is PV = c,
where P is pressure, V is volume and c is a constant. As-
15. Suppose that the average yearly cost per item for produc- sume that both P and V are functions of time. (a) Show that
ing x items of a business product is C(x) = 10 + 100 . If the P (t)∕V (t) =−c∕V . (b) Solve for P as a function of V. Treat-
′
′
2
x
current production is x = 10 and production is increasing ing V as an independent variable, compute P (V). Compare
′
at a rate of 2 items per year, find the rate of change of the P (V) and P (t)∕V (t) from parts (a) and (b).
′
′
′
average cost.
16. For a small company spending AED x thousand per year in 21. A dock is 6 ft above water. Suppose you stand on the edge of
advertising, suppose that annual sales in thousands of dol- the dock and pull a rope attached to a boat at the constant
lars equal s = 80 − 20e −0.04x . If the current advertising bud- rate of 2 ft/sec. Assume that the boat remains at water level.
get is x = 40 and the budget is increasing at a rate of AED At what speed is the boat approaching the dock when it is
1500 per year, find the rate of change of sales. 20 feet from the dock? 10 feet from the dock? Isn’t it sur-
17. A baseball player stands 2 feet from home plate and watches prising that the boat’s speed is not constant?
a pitch fly by. In the diagram, x is the distance from the ball 22. Sand is poured into a conical pile with the height of the pile
to home plate and is the angle indicating the direction of equalling the diameter of the pile. If the sand is poured at a
3
′
the player’s gaze. (a) Find the rate at which his eyes must constant rate of 5 m /s, at what rate is the height of the pile
′
move to watch a fastball with x (t) =−130 ft/s as it crosses increasing when the height is 2 meters?
home plate at x = 0. (b) Humans can maintain focus only 23. The frequency at which a guitar string vibrates (which deter-
′
when ≤ 3. Find the fastest pitch that you could actually mines the pitch of the note we hear) is related to the tension
watch cross home plate.
T to which the string is tightened, the density of the string
and the effective length L of the string by the equation
x
Plate 1 √ T
f = . By running his finger along a string, a guitarist
2L

θ 2 can change L by changing the distance between the bridge
Player √ T
and his finger. Suppose that L = 1 ft and = 220 ft/s so

2
18. A camera tracks the launch of a vertically ascending space- that the units of f are Hertz (cycles per second). If the gui-
craft. The camera is located at ground level 2 miles from tarist’s hand slides so that L (t) =−4, find f (t). At this rate,
′
′
the launchpad. (a) If the spacecraft is 3 miles up and trav- how long will it take to raise the pitch one octave (that is,
eling at 0.2 mile per second, at what rate is the camera an- double f)?
gle (measured from the horizontal) changing? (b) Repeat
if the spacecraft is 1 mile up (assume the same velocity). 24. Suppose that you are blowing up a balloon by adding air at
3
Which rate is higher? Explain in commonsense terms why the rate of 1 ft /s. If the balloon maintains a spherical shape,
4
3
it is larger. the volume and radius are related by V = r . Compare
3
the rate at which the radius is changing when r = 0.01 ft
versus when r = 0.1 ft. Discuss how this matches the expe-
rience of a person blowing up a balloon.
APPLICATIONS 25. Water is being pumped into a spherical tank of radius 60 feet
attheconstantrateof10ft /sec.(a)Findtherateatwhichthe
3
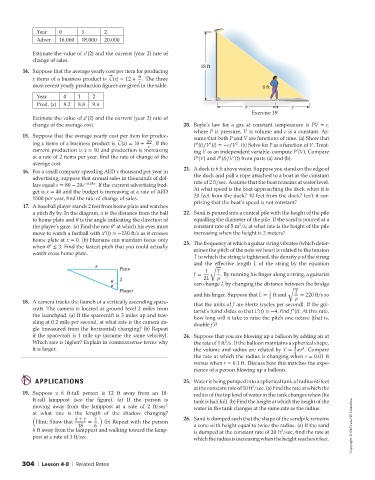
19. Suppose a 6 ft-tall person is 12 ft away from an 18- radius of the top level of water in the tank changes when the
ft-tall lamppost (see the figure). (a) If the person is tank is half full. (b) Find the height at which the height of the
moving away from the lamppost at a rate of 2 ft/sec 2 water in the tank changes at the same rate as the radius.
at what rate is the length of the shadow changing?
( x + s s ) 26. Sand is dumped such that the shape of the sandpile remains
Hint: Show that = . (b) Repeat with the person a cone with height equal to twice the radius. (a) If the sand
6
18
6 ft away from the lamppost and walking toward the lamp- is dumped at the constant rate of 20 ft /sec, find the rate at Copyright © McGraw-Hill Education
3
post at a rate of 3 ft/sec. whichtheradiusisincreasingwhentheheightreaches6feet.
304 | Lesson 4-8 | Related Rates