Page 101 - u4

P2: OSO/OVY
QC: OSO/OVY
T1: OSO
P1: OSO/OVY
UAE_Math_Grade_12_Vol_1_SE_718383_ch3
GO01962-Smith-v1.cls
July 4, 2016
13:38
1
and so, the only critical number is x = . Notice that the graph of y = f(x)isa
2
parabola opening downward and hence, the critical number must correspond to
the absolute maximum. Although the mathematical problem here was easy to
solve, the result gives a chemist some precise information. At the time the reaction
rate reaches a maximum, the concentration of chemical equals exactly half of the
saturation level.
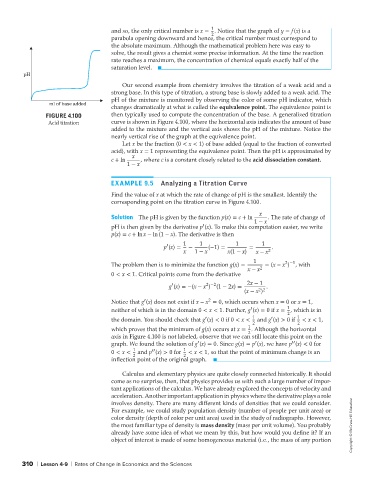
pH
Our second example from chemistry involves the titration of a weak acid and a
strong base. In this type of titration, a strong base is slowly added to a weak acid. The
pH of the mixture is monitored by observing the color of some pH indicator, which
ml of base added
changes dramatically at what is called the equivalence point. The equivalence point is
FIGURE 4.100 then typically used to compute the concentration of the base. A generalized titration
Acid titration curve is shown in Figure 4.100, where the horizontal axis indicates the amount of base
added to the mixture and the vertical axis shows the pH of the mixture. Notice the
nearly vertical rise of the graph at the equivalence point.
Let x be the fraction (0 < x < 1) of base added (equal to the fraction of converted
acid), with x = 1 representing the equivalence point. Then the pH is approximated by
c + ln x , where c is a constant closely related to the acid dissociation constant.
1 − x

EXAMPLE 9.5 Analyzing a Titration Curve

Find the value of x at which the rate of change of pH is the smallest. Identify the
corresponding point on the titration curve in Figure 4.100.

x
Solution The pH is given by the function p(x) = c + ln 1 − x . The rate of change of
′
pH is then given by the derivative p (x). To make this computation easier, we write
p(x) = c + ln x − ln (1 − x). The derivative is then
′
p (x) = 1 − 1 (−1) = 1 = 1 .
x 1 − x x(1 − x) x − x 2
1
2 −1
The problem then is to minimize the function g(x) = = (x − x ) , with
x − x 2
0 < x < 1. Critical points come from the derivative
2 −2
′
g (x) =−(x − x ) (1 − 2x) = 2x − 1 .
2 2
(x − x )
′
2
Notice that g (x) does not exist if x − x = 0, which occurs when x = 0 or x = 1,
1
′
neither of which is in the domain 0 < x < 1. Further, g (x) = 0 if x = , which is in
2
′
′
the domain. You should check that g (x) < 0if0 < x < 1 2 and g (x) > 0 if 1 2 < x < 1,
1
which proves that the minimum of g(x) occurs at x = . Although the horizontal
2
axis in Figure 4.100 is not labeled, observe that we can still locate this point on the
′′
′
′
graph. We found the solution of g (x) = 0. Since g(x) = p (x), we have p (x) < 0 for
′′
0 < x < 1 and p (x) > 0 for 1 < x < 1, so that the point of minimum change is an
2
2
inflection point of the original graph.
Calculus and elementary physics are quite closely connected historically. It should
come as no surprise, then, that physics provides us with such a large number of impor-
tant applications of the calculus. We have already explored the concepts of velocity and
acceleration. Another important application in physics where the derivative plays a role
involves density. There are many different kinds of densities that we could consider.
For example, we could study population density (number of people per unit area) or
color density (depth of color per unit area) used in the study of radiographs. However,
the most familiar type of density is mass density (mass per unit volume). You probably Copyright © McGraw-Hill Education
already have some idea of what we mean by this, but how would you define it? If an
object of interest is made of some homogeneous material (i.e., the mass of any portion
310 | Lesson 4-9 | Rates of Change in Economics and the Sciences