Page 99 - Mathematics Coursebook

9.1 Collecting like terms

9.1 Collecting like terms

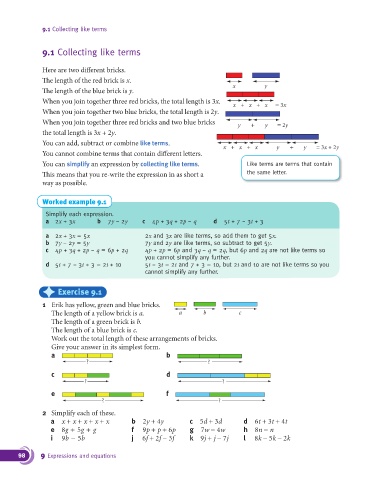
Here are two di#erent bricks.

x x y y

!e length of the red brick is x. x y
!e length of the blue brick is y. x y

When you join together three red bricks, the total length is 3x. x + x + x + x = 3x = 3x
x + x
x + x + x = 3x
When you join together two blue bricks, the total length is 2y. x + x + x = 3x
When you join together three red bricks and two blue bricks y y + + y y = 2y = 2y
the total length is 3x + 2y. y y + y y = 2y
= 2y
+
You can add, subtract or combine like terms.
x + x + x y + y = 3x + 2y
You cannot combine terms that contain di#erent letters. x + x + x y y + y y = 3x + 2y

x + x
+
= 3x + 2y
+ x
You can simplify an expression by collecting like terms. x + x + x y + y = 3x + 2y
Like terms are terms that contain
!is means that you re-write the expression in as short a the same letter.

way as possible.
Worked example 9.1
Simplify each expression.
a 2x + 3x b 7y − 2y c 4p + 3q + 2p − q d 5t + 7 − 3t + 3

a 2x + 3x = 5x 2x and 3x are like terms, so add them to get 5x.
b 7y − 2y = 5y 7y and 2y are like terms, so subtract to get 5y.
c 4p + 3q + 2p − q = 6p + 2q 4p + 2p = 6p and 3q − q = 2q, but 6p and 2q are not like terms so
you cannot simplify any further.
d 5t + 7 − 3t + 3 = 2t + 10 5t − 3t = 2t and 7 + 3 = 10, but 2t and 10 are not like terms so you
cannot simplify any further.

) Exercise 9.1

1 Erik has yellow, green and blue bricks.
The length of a yellow brick is a. a b c
The length of a green brick is b.
The length of a blue brick is c.
Work out the total length of these arrangements of bricks.
Give your answer in its simplest form.
a b
? ? ? ?
? ? ? ? ? ? ? ?
c d
? ? ? ? ? ? ? ? ? ? ? ?
e ? ? f ? ?
? ? ? ? ? ? ? ?

2 Simplify each of these.
a x + x + x + x + x b 2y + 4y c 5d + 3d d 6t + 3t + 4t
e 8g + 5g + g f 9p + p + 6p g 7w − 4w h 8n − n
i 9b − 5b j 6f + 2f − 3f k 9j + j − 7j l 8k − 5k − 2k

98 9 Expressions and equations