Page 330 - Linear Models for the Prediction of Animal Breeding Values 3rd Edition

Appendix D: Methods for

Obtaining Approximate Reliability

for Genetic Evaluations

D.1 Computing Approximate Reliabilities for an Animal Model

Presented below is a method published by Meyer (1989) for obtaining approximate
values of repeatability or reliability for genetic evaluations from an animal model and
has been used to estimate reliabilities in the national dairy evaluation system in
Canada. The reliability for each animal is derived from the corresponding diagonal
element in the MME, adjusting for selected off-diagonal coefficients. For instance, the
section of the coefficient matrix (C) pertaining to an animal i with parents f and m and
with a record in a subclass h of a major fixed effect as HYS could be represented as:

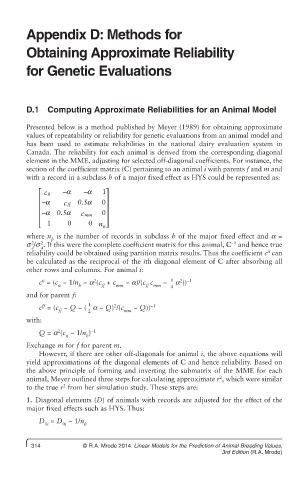
⎡ ii c -a -a 1⎤
⎢ ⎥
⎢ -a ff c 0.5a 0 ⎥
⎢ c mm 0⎥
⎢ -a 0.5a ⎥
⎣ 1 0 0 n h⎦

where n is the number of records in subclass h of the major fixed effect and a =
h
2
2
−1
s /s . If this were the complete coefficient matrix for this animal, C and hence true
e a
ii
reliability could be obtained using partition matrix results. Thus the coefficient c can
be calculated as the reciprocal of the ith diagonal element of C after absorbing all
other rows and columns. For animal i:
1
2
2
ii
c = (c − 1/n − a (c + c − a)/(c c − a )) −1
ii h ff mm ff mm 4
and for parent f:
1
ff
2
c = (c − Q − ( a − Q) /(c − Q)) −1
ff 2 mm
with:
2
Q = a (c − 1/n ) −1
ii h
Exchange m for f for parent m.
However, if there are other off-diagonals for animal i, the above equations will
yield approximations of the diagonal elements of C and hence reliability. Based on
the above principle of forming and inverting the submatrix of the MME for each
animal, Meyer outlined three steps for calculating approximate r , which were similar
2
2
to the true r from her simulation study. These steps are:
1. Diagonal elements (D) of animals with records are adjusted for the effect of the
major fixed effects such as HYS. Thus:
D = D − 1/n
1i 0i h
314 © R.A. Mrode 2014. Linear Models for the Prediction of Animal Breeding Values,
3rd Edition (R.A. Mrode)