Page 617 - Microeconomics, Fourth Edition

c14gametheoryandstrategicbehavior.qxd 8/6/10 8:22 AM Page 591

14.3 SEQUENTIAL-MOVE GAMES AND STRATEGIC MOVES 591

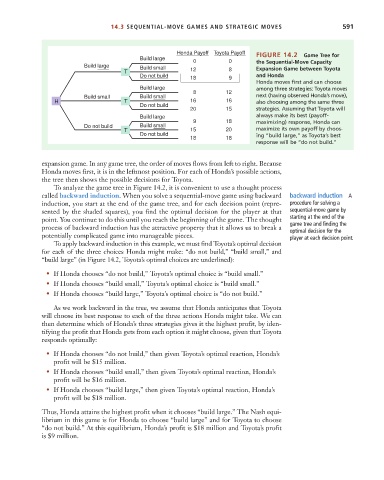
Honda Payoff Toyota Payoff FIGURE 14.2 Game Tree for
Build large
0 0 the Sequential-Move Capacity
Build large Build small Expansion Game between Toyota
T 12 8
Do not build 18 9 and Honda
Honda moves first and can choose
Build large among three strategies: Toyota moves
8 12
Build small Build small next (having observed Honda’s move),
H T 16 16 also choosing among the same three
Do not build
20 15 strategies. Assuming that Toyota will
Build large always make its best (payoff-
9 18 maximizing) response, Honda can
Do not build Build small
T 15 20 maximize its own payoff by choos-
Do not build ing “build large,” as Toyota’s best
18 18
response will be “do not build.”
expansion game. In any game tree, the order of moves flows from left to right. Because
Honda moves first, it is in the leftmost position. For each of Honda’s possible actions,
the tree then shows the possible decisions for Toyota.
To analyze the game tree in Figure 14.2, it is convenient to use a thought process
called backward induction. When you solve a sequential-move game using backward backward induction A
induction, you start at the end of the game tree, and for each decision point (repre- procedure for solving a
sented by the shaded squares), you find the optimal decision for the player at that sequential-move game by
point. You continue to do this until you reach the beginning of the game. The thought starting at the end of the
process of backward induction has the attractive property that it allows us to break a game tree and finding the
optimal decision for the
potentially complicated game into manageable pieces. player at each decision point.
To apply backward induction in this example, we must find Toyota’s optimal decision
for each of the three choices Honda might make: “do not build,” “build small,” and
“build large” (in Figure 14.2, Toyota’s optimal choices are underlined):

• If Honda chooses “do not build,” Toyota’s optimal choice is “build small.”
• If Honda chooses “build small,” Toyota’s optimal choice is “build small.”
• If Honda chooses “build large,” Toyota’s optimal choice is “do not build.”
As we work backward in the tree, we assume that Honda anticipates that Toyota
will choose its best response to each of the three actions Honda might take. We can
then determine which of Honda’s three strategies gives it the highest profit, by iden-
tifying the profit that Honda gets from each option it might choose, given that Toyota
responds optimally:

• If Honda chooses “do not build,” then given Toyota’s optimal reaction, Honda’s
profit will be $15 million.
• If Honda chooses “build small,” then given Toyota’s optimal reaction, Honda’s
profit will be $16 million.
• If Honda chooses “build large,” then given Toyota’s optimal reaction, Honda’s
profit will be $18 million.

Thus, Honda attains the highest profit when it chooses “build large.” The Nash equi-
librium in this game is for Honda to choose “build large” and for Toyota to choose
“do not build.” At this equilibrium, Honda’s profit is $18 million and Toyota’s profit
is $9 million.