Page 36 - Fiber Optic Communications Fund

Electromagnetics and Optics 17

If we take the real part of Eq. (1.101), we find
[ ]
̃
̃
Re E E = A A cos [( + )t −(k + k )z]
1
2
1 2
2
x1 x1
1
≠ E E . (1.102)
x1 x2
In this case, we should use the real form of electromagnetic fields. In the rest of this book we sometimes omit ̃
and use E (H ) to represent a complex electric (magnetic) field with the understanding that the real part is the
x y
actual field.
1.7 Power Flow and Poynting Vector
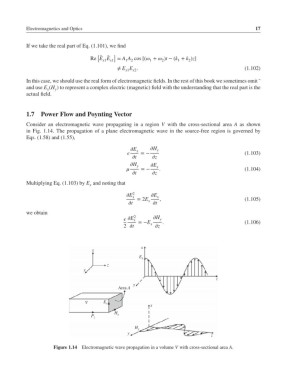
Consider an electromagnetic wave propagating in a region V with the cross-sectional area A as shown
in Fig. 1.14. The propagation of a plane electromagnetic wave in the source-free region is governed by
Eqs. (1.58) and (1.55),

E x H y
=− (1.103)
t z
H y E x
=− . (1.104)
t z

Multiplying Eq. (1.103) by E and noting that
x
E x 2 E x
= 2E x , (1.105)
t t
we obtain
E 2 x H y
=−E x . (1.106)
2 t z

x
x
E x
z
y
z
y
Area A

V E x
x
H y
P z
H y
y
z
Figure 1.14 Electromagnetic wave propagation in a volume V with cross-sectional area A.