Page 41 - Fiber Optic Communications Fund

22 Fiber Optic Communications

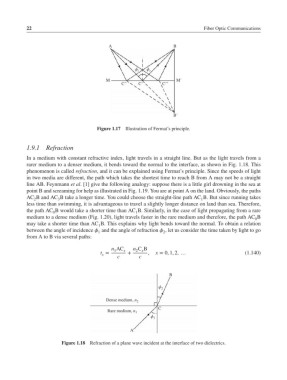
A B

ϕ i ϕ r
M Mʹ
Cʹʹ Cʹ Cʹʹʹ

Bʹ

Figure 1.17 Illustration of Fermat’s principle.

1.9.1 Refraction

In a medium with constant refractive index, light travels in a straight line. But as the light travels from a
rarer medium to a denser medium, it bends toward the normal to the interface, as shown in Fig. 1.18. This
phenomenon is called refraction, and it can be explained using Fermat’s principle. Since the speeds of light
in two media are different, the path which takes the shortest time to reach B from A may not be a straight
line AB. Feynmann et al. [1] give the following analogy: suppose there is a little girl drowning in the sea at
point B and screaming for help as illustrated in Fig. 1.19. You are at point A on the land. Obviously, the paths
AC B and AC B take a longer time. You could choose the straight-line path AC B. But since running takes
3
1
2
less time than swimming, it is advantageous to travel a slightly longer distance on land than sea. Therefore,
the path AC B would take a shorter time than AC B. Similarly, in the case of light propagating from a rare
0 1
medium to a dense medium (Fig. 1.20), light travels faster in the rare medium and therefore, the path AC B
0
may take a shorter time than AC B. This explains why light bends toward the normal. To obtain a relation
1
between the angle of incidence and the angle of refraction , let us consider the time taken by light to go
1 2
from A to B via several paths:
n AC x n C B
2 x
1
t = + , x = 0, 1, 2, … (1.140)
x
c c
B
ϕ 2

Dense medium, n 2
C
Rare medium, n 1
ϕ 1
A

Figure 1.18 Refraction of a plane wave incident at the interface of two dielectrics.